sin x cos y formula

sin x cos y formula Cosx cosy cos x y cos x y 2 sinx siny cos x y cos x y 2 Sum to Product Formulas The combination of two acute angles A and B can be presented through the trigonometric ratios in the below trigonometry formulas sinx siny 2 sin x y 2 cos x y 2 sinx siny 2 cos x y 2 sin x y 2

Power Reducing Formulas sin 2 X 1 2 1 2 cos 2X cos 2 X 1 2 1 2 cos 2X sin 3 X 3 4 sinX 1 4 sin 3X cos 3 X 3 4 cosX 1 4 cos 3X sin 4 X 3 8 1 2 cos 2X 1 8 cos 4X cos 4 X 3 8 1 2 cos 2X 1 8 cos 4X sin 5 X 5 8 sinX 5 16 sin 3X 1 16 sin 5X cos 5 X 5 8 cosX 5 16 cos 3X Angle sum and difference identities Double Angle Formulas Triple Angle Formulas Half Angle Identities Power reducing formulas Sum Identities Sum to Product Identities Product Identities Product to Sum Identities Law of sine Law of cosine What are Inverse Trigonometry Functions Domain and range of Inverse Trigonometry Functions

sin x cos y formula

sin x cos y formula
https://i.ytimg.com/vi/z6iPWPCoGOE/maxresdefault.jpg

Sin X Cos X 1 2 Find Value Of X YouTube
https://i.ytimg.com/vi/tIh11N7vNts/maxresdefault.jpg

Ex 3 3 18 Prove Sin X Sin Y cos X Cos Y Tan x Y 2
https://d1avenlh0i1xmr.cloudfront.net/d606ed1f-ea78-44bb-b50c-948c70a725d8slide21.jpg

Calculus Trigonometric substitution Integrals inverse functions Derivatives v t e In trigonometry trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of Thus sin Y 7 4 cos X 3 2 and sinY 7 4 Applying the sum of cos formula we have cos X Y 3 2 3 4 1 2 7 4 3 3 7 8 Answer cos X Y 3 3 7 8 Example 2 If sin 3 5 find sin2 Solution We know that sin2 2 sin cos We need to determine cos Let us use the sin cos

Cos x cos y 2 sin x y 2 sin x y 2 Given Triangle abc with angles A B C a is opposite to A b opposite B c opposite C a sin A b sin B c sin C Law of Sines c 2 a 2 b 2 2ab cos C b 2 a 2 c 2 2ac cos B a 2 b 2 c 2 2bc cos A Law of Cosines Write the formula for the product of cosines Substitute the given angles into the formula Simplify Example 3 4 1 3 4 1 Writing the Product as a Sum Using the Product to Sum Formula for Cosine Write the following product of cosines as a sum 2 cos 7x 2 cos 3x 2 2 cos 7 x 2 cos 3 x 2

More picture related to sin x cos y formula

10 3 Integration Of Trigonometric Functions
http://www.phengkimving.com/calc_of_one_real_var/10_techniques_of_intgrtn/10_03_intgrtn_of_trig_func_files/image043.gif

Misc 4 Prove cos X Cos Y 2 sin X Sin Y 2 Chapter 3
https://d1avenlh0i1xmr.cloudfront.net/2102d8a3-2a2c-44a5-a3de-e75ef7228d1aslide5.jpg

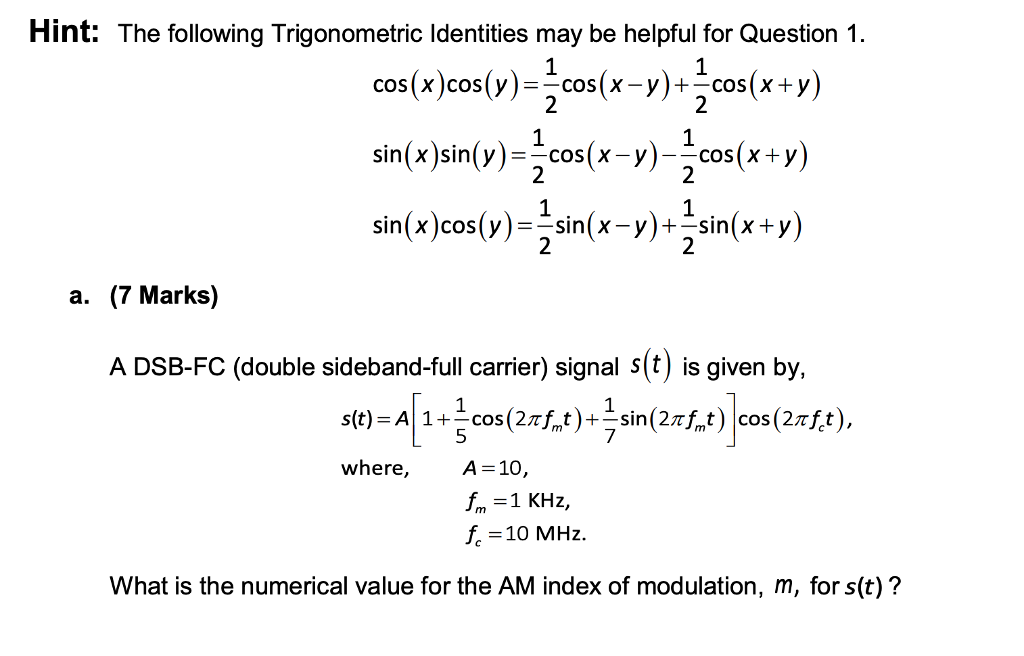
Solved Hint The Following Trigonometric Identities May Be Chegg
https://media.cheggcdn.com/media/4ad/4ad04e87-eead-4906-8d9f-b2401e4c19f3/phpzZHVDA.png

Consider the proof for the equation sin x sin y implies x n 1 n y where n Z and x and y are any real numbers If sin x sin y then sin x sin y 0 i e 2 cos x y 2 sin x y 2 0 using formula sin x y sin x cos y cos x sin y sin 11 12 sin 2 3 4 sin 2 3 cos 4 cos 2 3 sin 4 Sin 2 x cos x Cos 2 x sin x Sin 2 x cos x Cos 2 x sin x Sin 3 2 x cos x cos 3 2 x sin x Sin 3 2 x cos x Cos 3 2 x sin x Sin x sin x Cos x cos x Sin x sin x Cos x cos x Sin 2 x sin x

[desc-10] [desc-11]

Integral Of sin x Cos x sin x Cos x substitution YouTube
https://i.ytimg.com/vi/_8oCZ_UM2JQ/maxresdefault.jpg

D couvrir 112 Imagen Cos Sin Formule Fr thptnganamst edu vn
https://d1avenlh0i1xmr.cloudfront.net/867feefc-c46d-450d-8da3-f236ad6d5e0c/sum-identities-(sum-to-product-identities).jpg

sin x cos y formula - Calculus Trigonometric substitution Integrals inverse functions Derivatives v t e In trigonometry trigonometric identities are equalities that involve trigonometric functions and are true for every value of the occurring variables for which both sides of