how to find d dx Calculate the Derivative of CLR This will be calculated d dx sin ex a 2 Not what you mean Use parentheses Set differentiation variable and order in Options Support Donate Amazon Result Above enter the function to derive Differentiation variable and more can be changed in Options
frac d dx frac 3x 9 2 x frac d 2 dx 2 frac 3x 9 2 x sin 2 theta derivative of f x 3 4x 2 x 5 implicit derivative frac dy dx x y 2 x y 1 Example x 2 y 2 r 2 Differentiate with respect to x d dx x 2 d dx y 2 d dx r 2 Let s solve each term Use the Power Rule d dx x2 2x Use the Chain Rule explained below d dx y2 2y dy dx r 2 is a constant so its derivative is 0 d dx r2 0 Which gives us 2x 2y dy dx 0
how to find d dx

how to find d dx
https://i.ytimg.com/vi/suLE9yJ9FRY/maxresdefault.jpg
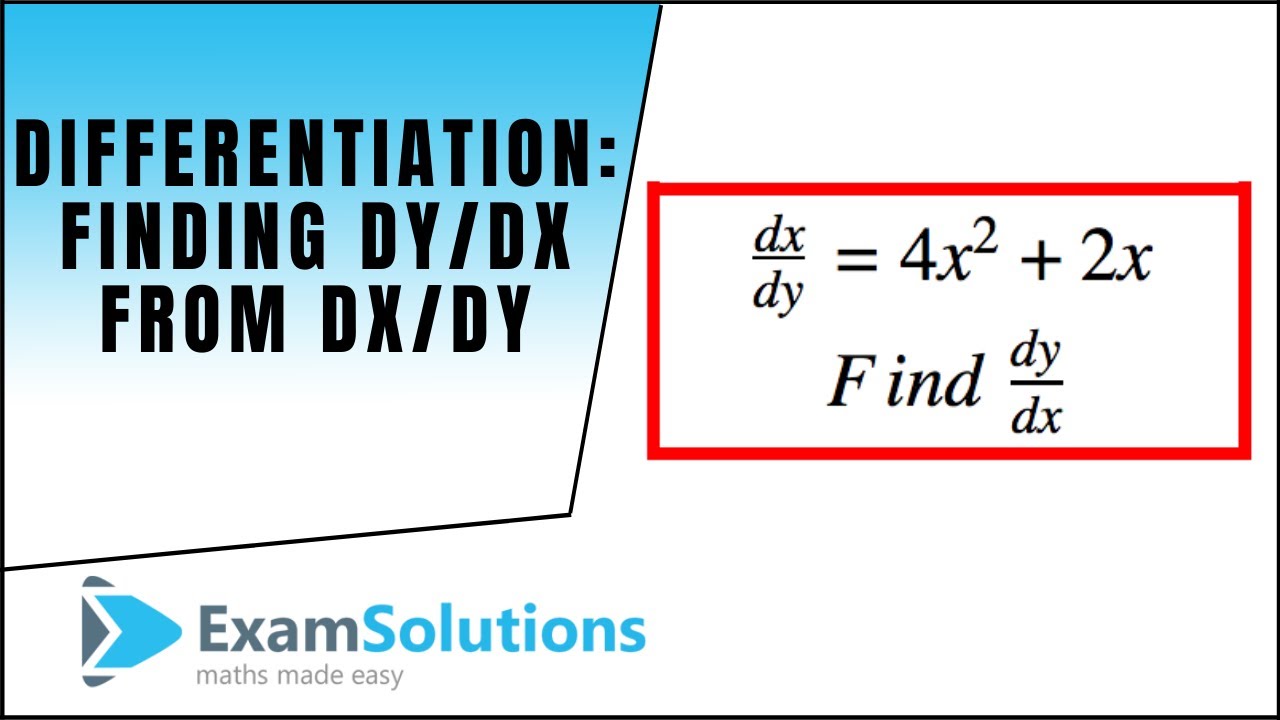
Differentiation Finding Dy dx From Dx dy Example 1 ExamSolutions
https://i.ytimg.com/vi/Mja823c2ENs/maxresdefault.jpg

Find Dy dx Implicit Differentiation YouTube
https://i.ytimg.com/vi/VL-rePJAjaY/maxresdefault.jpg
For example x y 1 Implicit differentiation helps us find dy dx even for relationships like that This is done using the chain rule and viewing y as an implicit function of x For example according to the chain rule the derivative Try It On A Function Let s try f x x 2 dy dx f x dx f x dx Use f x x 2 x dx 2 x2 dx Expand x dx 2 x2 2x dx dx 2 x2 dx x 2 x 2 0 2x dx dx 2 dx Simplify fraction 2x dx dx goes towards 0 2x So the derivative of x2 is 2x Why don t you try it on f x x 3
7 75M subscribers Subscribed 9 6K 634K views 5 years ago This calculus video tutorial provides a basic introduction into implicit differentiation it explains how to find the first derivative Take the derivative of both sides of the equation Keep in mind that y is a function of x Consequently whereas dfrac d dx sin x cos x nonumber and dfrac d dx sin y cos y cdot dfrac dy dx nonumber because we must use the chain rule to differentiate sin y with respect to x
More picture related to how to find d dx
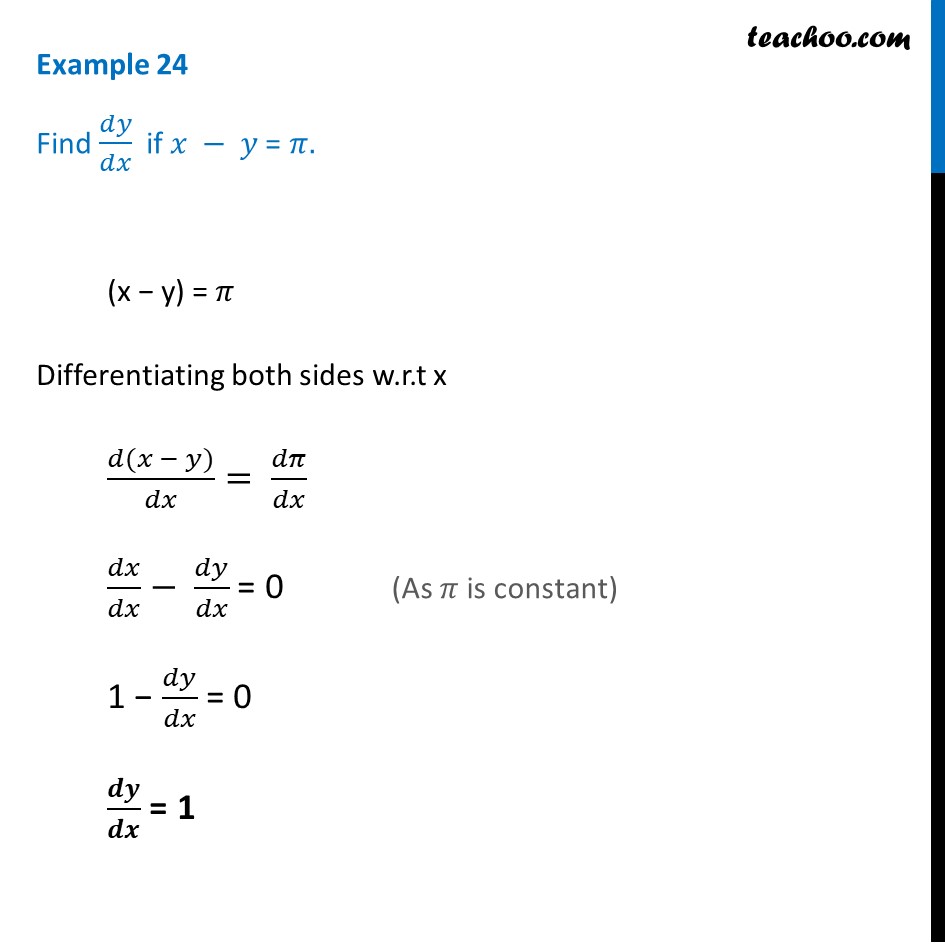
Example 24 Find Dy dx If X Y Pi Chapter 5 NCERT Examples
https://d1avenlh0i1xmr.cloudfront.net/4aaa8faa-7e94-4b5f-a152-13adf34b5bf8/slide57.jpg

Ex 9 4 14 Find Particular Solution Dy dx Y Tan X Y 1
https://d1avenlh0i1xmr.cloudfront.net/large/df858407-652b-4e17-b549-f9f776384c2c/slide8.jpg

Ex 5 6 5 Find Dy dx X Cos Cos 2 Y Sin Sin 2
https://d1avenlh0i1xmr.cloudfront.net/a0d2c343-468a-41be-9ba0-acb4acec0721/slide9.jpg
Home Bookshelves Calculus 3e Apex 2 Derivatives 2 6 Implicit Differentiation Expand collapse global location 2 6 Implicit Differentiation Page ID Gregory Hartman et al Virginia Military Institute In the previous sections we learned to find the derivative dy dx or y when y is given explicitly as a function of x D dx x 1 2 1 Find the derivative of the outside Consider the outside 2 as x 2 and find the derivative as d dx x 2 2x the outside portion 2 2 Add the inside into the parenthesis 2 2 x 1 3 Find the derivative of the inside and multiply as d dx x 1 1 1 2 x 1 2 x 1 Thus d dx x 1 2 2 x 1
It is pronounced as Dee by dee ex Defining d dx While studying differential equations you will come across d dx vs dy dx So what is the difference between these two terms If we write d d x as d y d x then this means that we are differentiating dependent variable y with respect to independent variable x Derivative Calculator Step 1 Enter the function you want to find the derivative of in the editor The Derivative Calculator supports solving first second fourth derivatives as well as implicit differentiation and finding the zeros roots
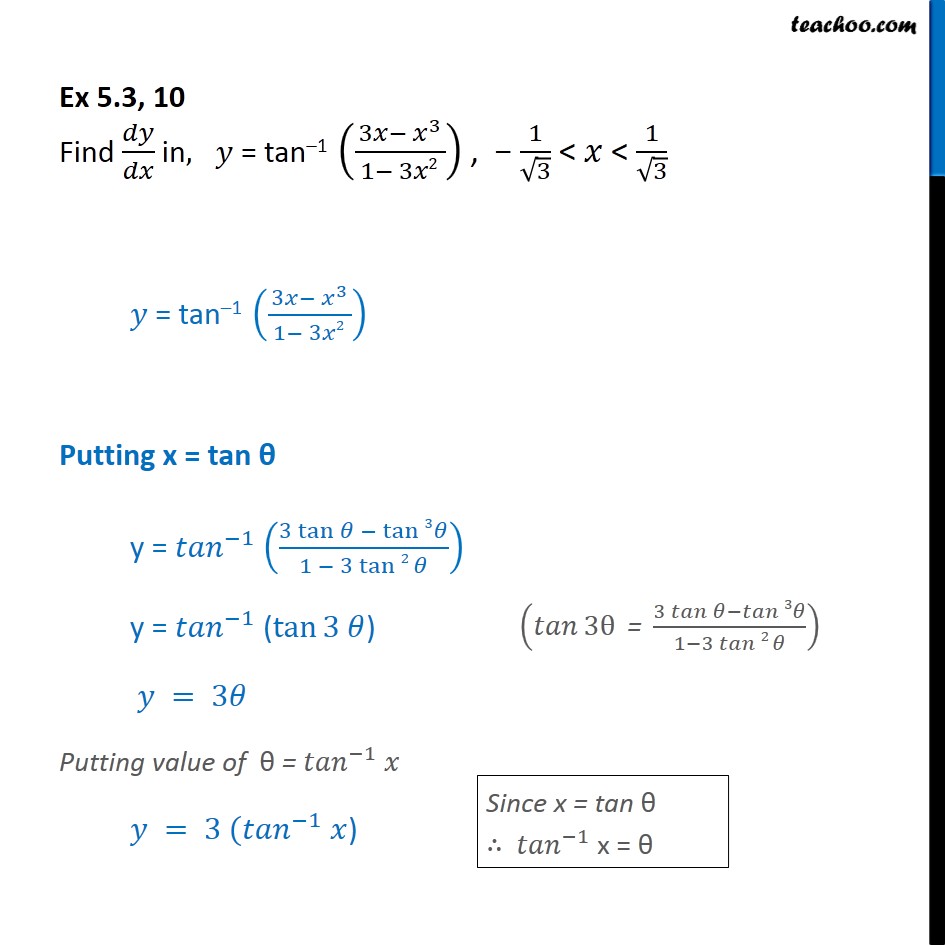
Ex 5 3 10 Find Dy dx In Y tan 1 3x X3 1 3x2 Ex 5 3
https://d77da31580fbc8944c00-52b01ccbcfe56047120eec75d9cb2cbd.ssl.cf6.rackcdn.com/f24702b2-df18-4ed2-a86f-49644ec222b9/slide21.jpg

Solve The Differential Equation Tanx dy Coty dx YouTube
https://i.ytimg.com/vi/90-ZANbnosQ/maxresdefault.jpg
how to find d dx - Take the derivative of both sides of the equation Keep in mind that y is a function of x Consequently whereas dfrac d dx sin x cos x nonumber and dfrac d dx sin y cos y cdot dfrac dy dx nonumber because we must use the chain rule to differentiate sin y with respect to x