how does a system have infinite solutions So when does a system of linear equations have infinite solutions A system of two linear equations in two variables has infinite solutions if the two lines are the same From an algebra standpoint we get an equation that is always true if we solve the system
The system of an equation has infinitely many solutions when the lines are coincident and they have the same y intercept If the two lines have the same y intercept and the slope they are actually in the same exact line After solving for y I get an equation say K x C where K is coefficeint of x and C is a constant Then the equations have 1 No solutions if K is same but C is different in both equation 2 infinite solutions if K and C are equal for both equations 3 and one solution if K is different Is this right
how does a system have infinite solutions

how does a system have infinite solutions
https://media.nagwa.com/384163156847/en/thumbnail_l.jpeg

How To Solve A System Of Equations With Infinite Many Solutions YouTube
https://i.ytimg.com/vi/C6Z3GCB2JMM/maxresdefault.jpg
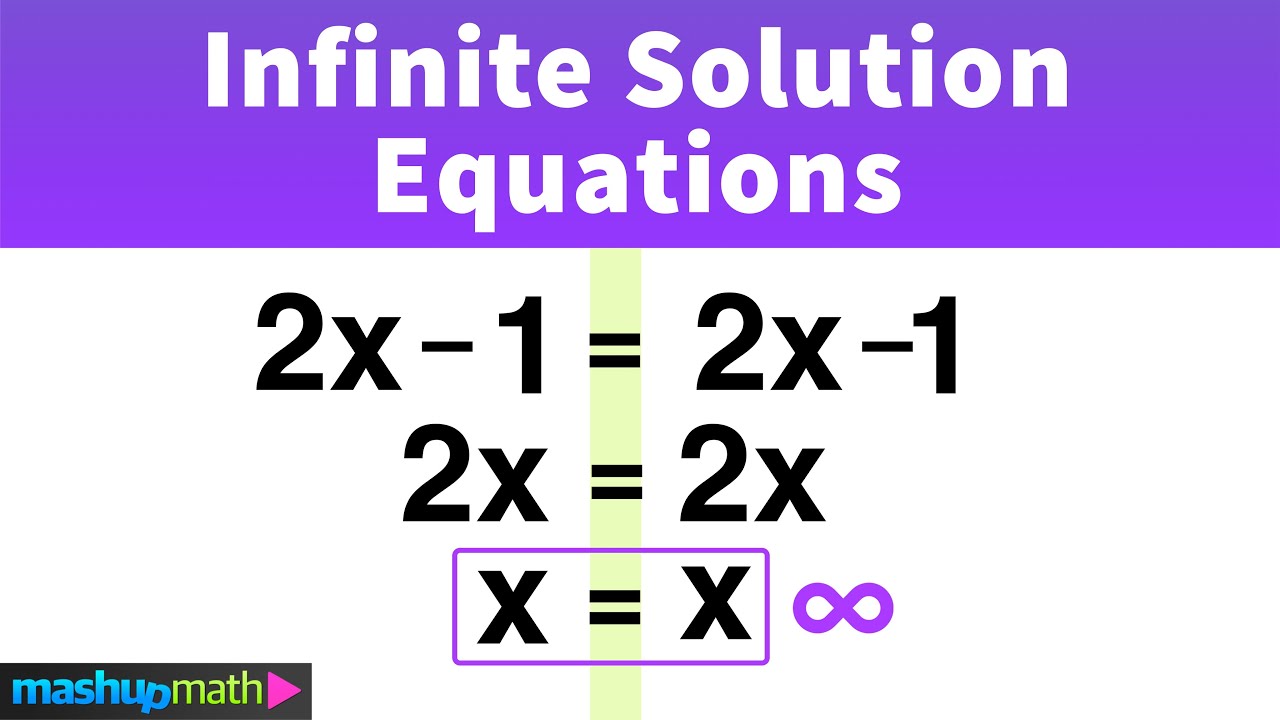
Infinite Solutions Equations Explained YouTube
https://i.ytimg.com/vi/o_zob5jIn6E/maxresdefault.jpg
If a consistent linear system of equations has a free variable it has infinite solutions If a consistent linear system has more variables than leading 1s then the system will have infinite solutions A consistent linear system with more variables than equations will always have infinite solutions No If the matrix is singular i e if det A 0 the system has 0 solution or an infinity of solutions The general criterion is based on the augmented matrix A B Let A be an m times n matrix r its rank B an m times 1 matrix The system of equations AX B has a solution if and only if operatorname rank A operatorname
Nope In a system of equations the solution is the point where the graphed lines intersect Parallel lines never intersect so they have zero solutions Lines that are the same are always together so those are the ones with infinite solutions So we have a system of equations that are linear d 0 2t d 0 5 t 6 We can solve it on a graph One or infinitely many solutions are called consistent Here is a diagram for 2 equations in 2 variables Independent Independent means that
More picture related to how does a system have infinite solutions

Solve A System Of Equations By Graphing ALL 3 CASES One No Or
https://i.ytimg.com/vi/e8_wlEh4mBY/maxresdefault.jpg

One Solution Infinite Solutions No Solutions Guided Notes Video YouTube
https://i.ytimg.com/vi/TBRCrGBGwxs/maxresdefault.jpg
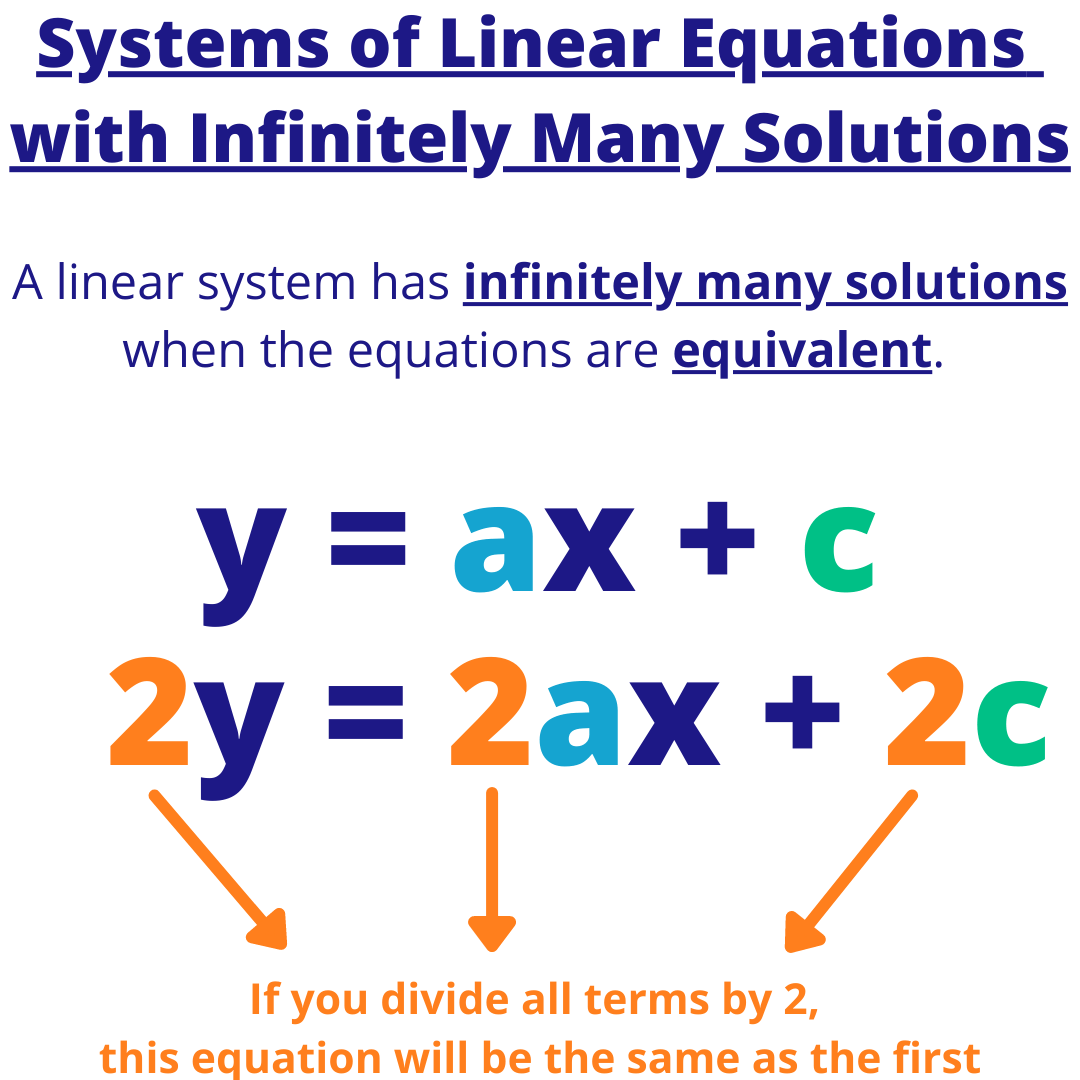
Systems Of Linear Equations With Infinite Solutions Examples Expii
https://d20khd7ddkh5ls.cloudfront.net/systems_of_linear_equations_with_infinitely_many_solutions.png
How can you determine if a linear system has infinitely many solutions directly from its reduced row echelon matrix What can you say the solution space of a linear system if there are more unknowns than equations and at least one solution exists How can systems have infinite solutions If you solve a system with graphing you might notice that the solution is where the lines cross Let s look at a system of equations that has infinite solutions 22x 11y 4414x 7y 28 Try simplifying the top equation What do you notice 2x y 42x y 4 These are same equation
Reconize when a matrix has a unique solutions no solutions or infinitely many solutions using python Unique Solution The example shown previously in this module had a unique solution The structure of the row reduced matrix was 1 0 0 1 1 0 1 5 1 5 8 1 1 1 1 5 0 1 5 8 0 0 1 1 and the solution was Can a system of equations have more than one but not infinite number of solutions No you can only have either a unique solution or infinite solutions Let us suppose lbrack A rbrack lbrack X rbrack lbrack C rbrack has two solutions lbrack Y rbrack and lbrack Z rbrack so that
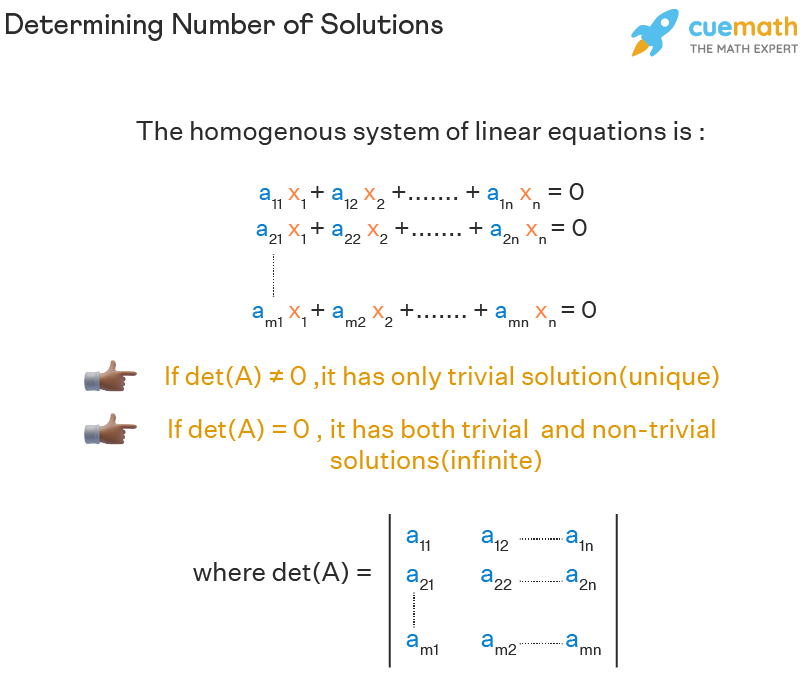
Homogeneous System Of Linear Equations Solution Examples
https://d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/homogenous-system-of-linear-equations-formula-1641887965.png

Solving A System With Infinitely Many Solutions YouTube
https://i.ytimg.com/vi/OA4YDDH0xvQ/maxresdefault.jpg
how does a system have infinite solutions - About Transcript A dependent system of equations has infinite solutions and an independent system has a single solution Watch an example of analyzing a system to see if it s dependent or independent Created by Sal Khan and Monterey Institute for Technology and Education Questions Tips Thanks Want to join the conversation Log in Sort by