cos2x sin2x identity Basic trig identities are formulas for angle sums differences products and quotients and they let you find exact values for trig expressions
Sin 2x cos 2x is one of the trigonometric identities which is essential for solving a variety of trigonometry related questions Here the simplified value of Sin2x cos2x is given along with the integral and derivative of sin2x and cos 2x The sin 2x formula is the double angle identity used for the sine function in trigonometry It is sin 2x 2sinxcosx and sin 2x 2tan x 1 tan 2x On the other hand sin 2x identities are sin 2x 1 cos 2x and sin 2x 1 cos 2x 2
cos2x sin2x identity

cos2x sin2x identity
https://i0.wp.com/drumconclusions.com/0213332e/https/dc1982/d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/cos-2x-identity-1628687983.png

Trigonometric Identity With Double Angle Formula Sin2x Cos 2x YouTube
https://i.ytimg.com/vi/jYbt9fT9a00/maxresdefault.jpg

Membahas Tentang Sin 2X Cos 2X Wanjay
https://i2.wp.com/www.petervis.com/mathematics/integration-solutions/integrate-sin_2_x-cos_2_x/step-02.gif
Pythagoras theorem Given a right angled triangle with sides a b and c consider the following diagram The area of the large square is a b 2 The area of the small tilted square is c2 The area of each triangle is 1 2 ab So we have a b 2 c2 4 1 2 ab That is a2 2ab b2 c2 2ab Derivation of Sin 2x Cos 2x We make use of the trigonometry double angle formulas to derive this identity We know that sin 2x 2 sin x cos x i cos 2x cos2 x sin2 x 2 cos2 x 1 because sin2x cos2 x 1 ii 1 2 Sin2x iii We want to find the value of sin
In this section we will begin an examination of the fundamental trigonometric identities including how we can verify them and how we can use them to simplify trigonometric expressions Trigonometric identities like sin cos 1 can be used to rewrite expressions in a different more convenient way For example 1 sin cos can be rewritten as cos cos and then as cos
More picture related to cos2x sin2x identity
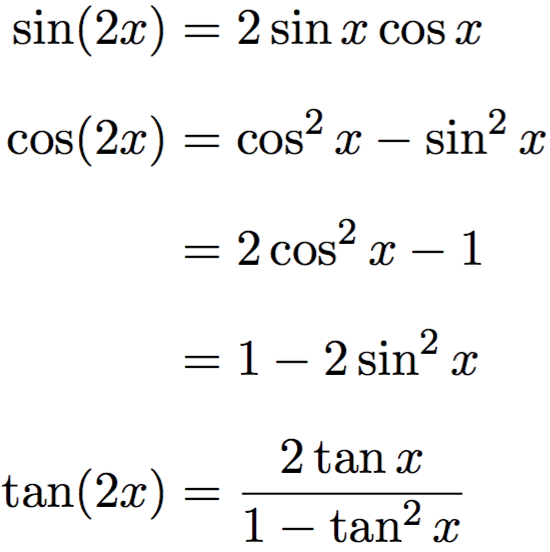
Basic Trigonometric Identities
https://evgenii.com/image/blog/2016-05-18-basic-trigonometric-identities/double_angles_trigonometry.png
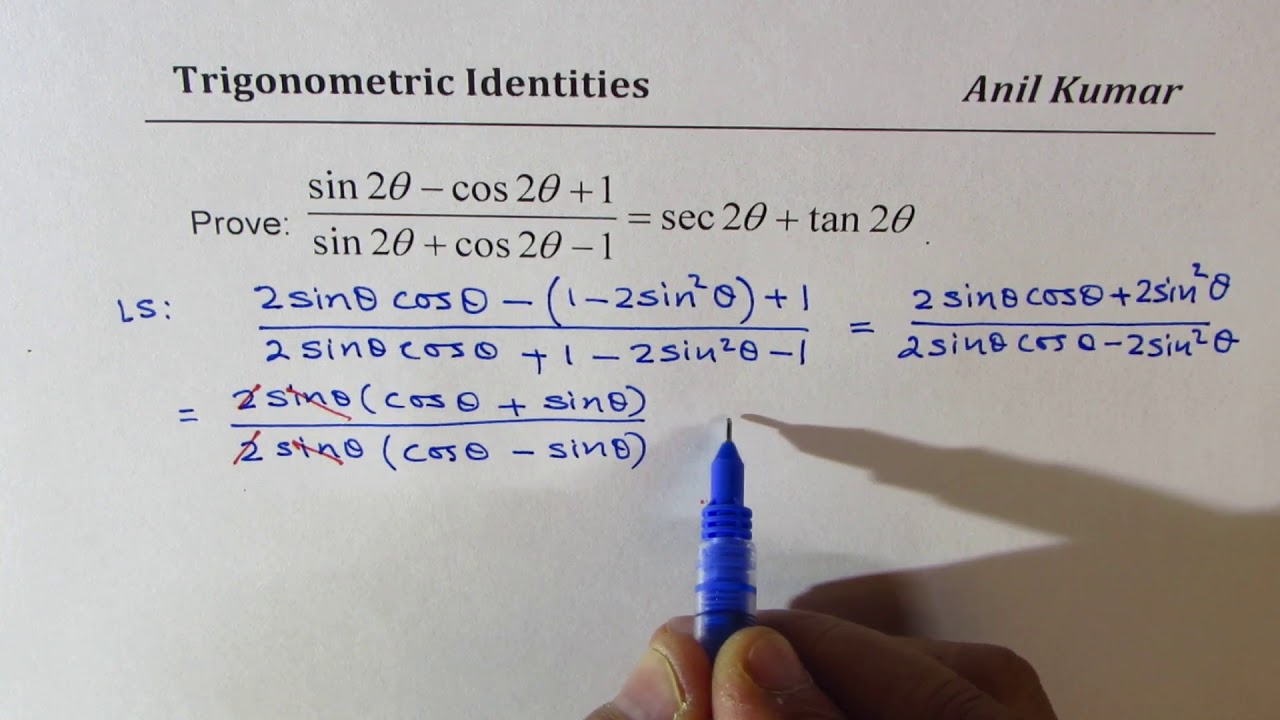
Trigonometric Identity In Double Angle sin2x Cos2x 1 sin2x
https://i.ytimg.com/vi/XrbDRmcREPc/maxresdefault.jpg
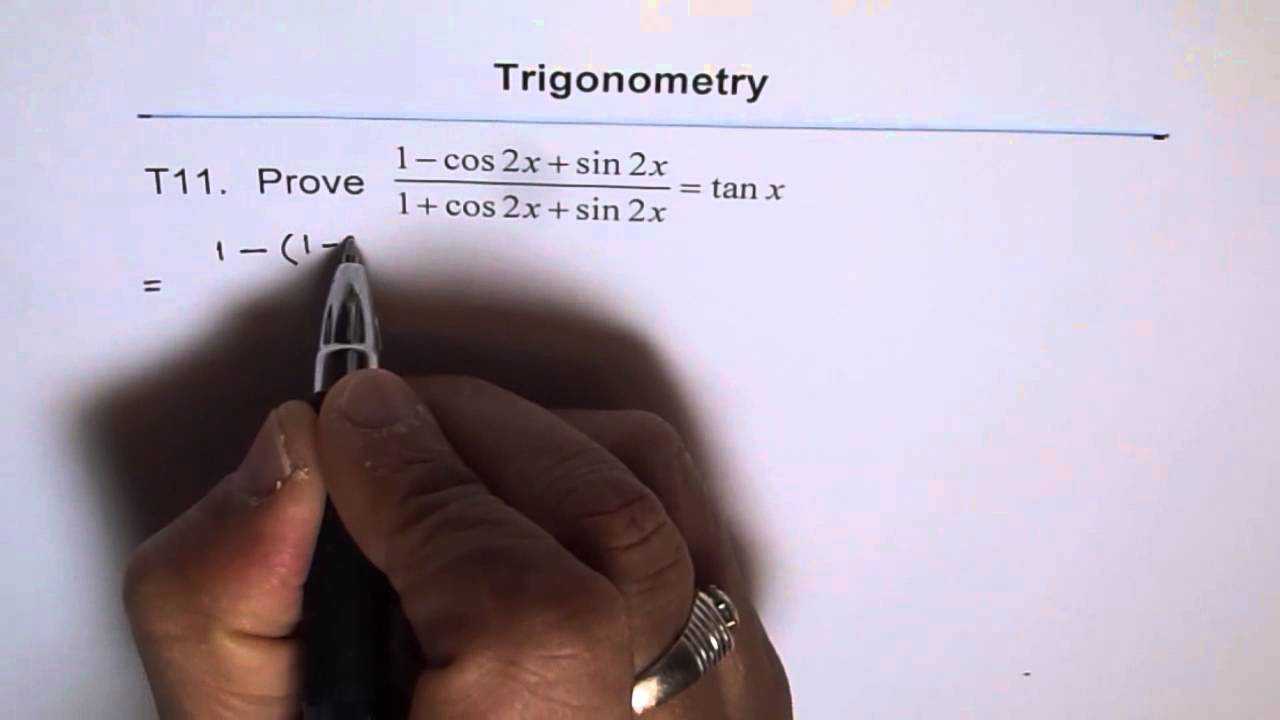
Trigonometric Identity 1 Cos2x Sin2x 1 Cos2x Sin2x Tanx
https://i.ytimg.com/vi/ay0lRNVd2LA/maxresdefault.jpg
How do you use the fundamental identities to prove other identities Divide the fundamental identity sin 2x cos 2x 1 by sin 2x or cos 2x to derive the other two sin 2x sin 2x cos 2x sin 2x 1 sin 2x Using the cosine double angle identity The cosine double angle formula tells us that cos 2 is always equal to cos sin For example cos 60 is equal to cos 30 sin 30 We can use this identity to rewrite expressions or solve
[desc-10] [desc-11]
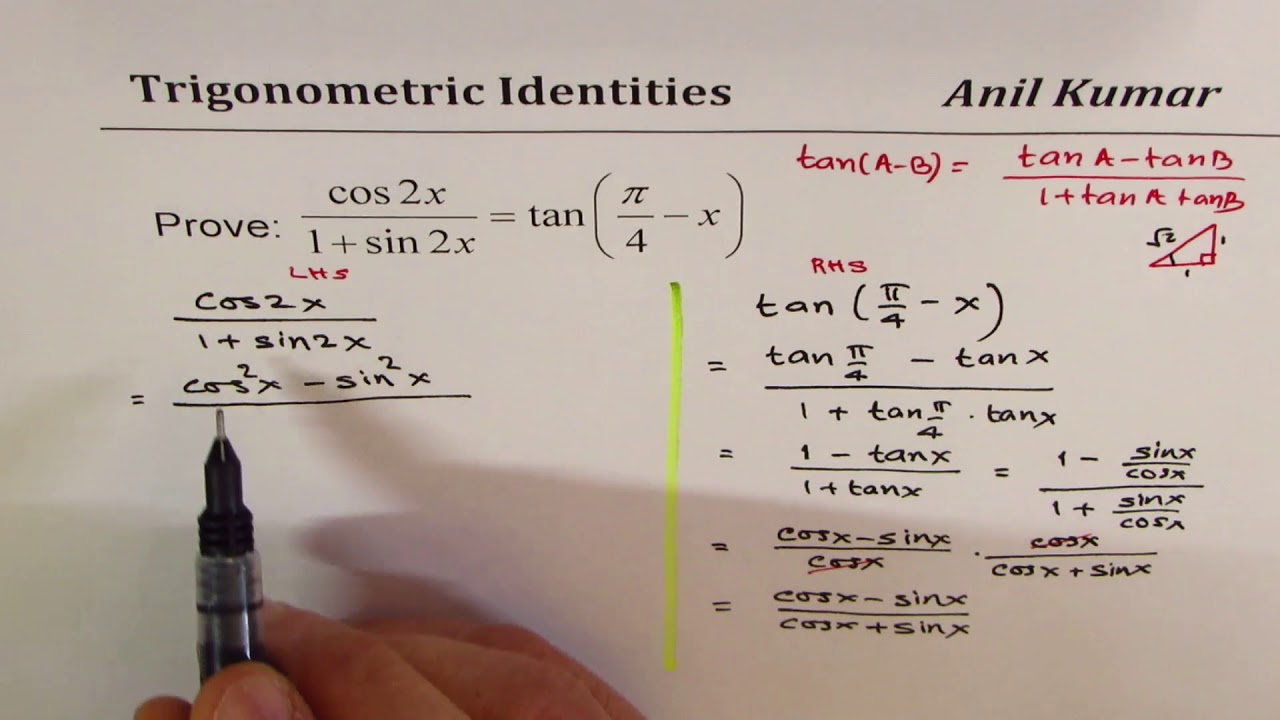
Cos2x 1 Sin2x Tan pi 4 X Double And Half Angle Identity YouTube
https://i.ytimg.com/vi/pv2YY1oa0w0/maxresdefault.jpg
MATH 1316 Section 5 5 Solving For Cos2x And Sin2x Values Using Double
https://i.ytimg.com/vi/MAOJUPrL1zQ/maxresdefault.jpg
cos2x sin2x identity - [desc-14]