basic log rules pdf The first law of logarithms loga xy loga x loga y 4 4 6 The second law of logarithms loga xm m loga x 5 7 The third law of logarithms loga x loga x loga y 5 1 Introduction In this unit we are going to be looking at logarithms However before we can deal with logarithms we need to revise indices
Log is often written as e x ln x and is called the NATURAL logarithm note e 2 7182818284 59 PROPERTIES OF LOGARITHMS EXAMPLES 1 log b MN log b M log b N log 50 log 2 log 100 2 Think Multiply two numbers with the same base add the exponents 2 M N N M log b log b log b log 8 1 7 56 log If either a 1 or 0 a 1 then the inverse of the function ax is loga 0 1 and it s called a logarithm of base a That ax and loga x are inverse functions means that aloga x x and loga ax x Problem Find x if 2x 15 Solution The inverse of an exponential function with base 2 is log2
basic log rules pdf

basic log rules pdf
https://www.publicdomainpictures.net/pictures/30000/velka/log.jpg

Log Rules Narural Log Rules Rules Of Ln Logarithm Rules
https://d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/log-or-logarithm-rules-1647317216.png

Logarithm Rules Study Pivot 2 Medium
https://cdn-images-1.medium.com/max/1600/1*AvjkLYUMrXaJNNMAmlotiw.png
Typically today s students experience teachers incanting The log of a product is the sum of the logs The log of a quotient is the difference of the logs The students see the rules with little development of ideas behind them or history of how they were used in conjunction with log Y 16 Now take it out of the exponential form and write it in logarithmic form Just like 23 8 converts to log 8 3 2 Ask your teacher about the last two examples They may show you a nice shortcut Finally we want to take a look at the Property of Equality for Logarithmic Functions Supp ose b 0 and b 1
Using this de nition we can check that rules 1 and 3 also remain valid For example to check that rule 1 still holds if n is a whole number and m 0 then rule 1 gives bn b0 bn which is okay because b0 1 To be strictly correct we should also check that rule 1 remains valid in the case that m 0 and n 0 You should check Section 1 Logarithms 3 1 Logarithms Introduction Let aand N be positive real numbers and let N an Then nis called the logarithm of Nto the base a We write this as n log a N Examples 1 a Since 16 24 then 4 log 216 b Since 81 34 then 4 log 381 c Since 3 p 9 91 2 then 1 2 log 93 d Since 31
More picture related to basic log rules pdf
Log Rules Yup Math
https://d33wubrfki0l68.cloudfront.net/4c93c5b12da8488fa19ba04718426a72b3377c97/19145/ef925d0850547459e95bbfced9fe7b36/log_rules.svg

Rules Of The Logarithms logarithms rules Algebra Math Formulas
https://i.pinimg.com/originals/82/8b/8d/828b8deb9c2a1a743e5f9ae4a97dedc0.jpg

Laws Of Logarithms InertiaLearning
https://www.inertialearning.com/wp-content/uploads/2021/05/logarithm-rules.png
Rules of Exponentials and Logarithms Let a m and n be real numbers The following rules hold for any log c x c 0 but are presented using the natural log function loge x ln x as we will use this most often Let a and b be real numbers 0 In general log c x is unde ned for x 0 The laws of logarithms The three main laws are stated here First Law log A log B log AB This law tells us how to add two logarithms together Adding log A and log B results in the logarithm of the product of A and B that is log AB For example we can write log 6 log 2 log 10 6 2 log 10 10 10 12
Introduction to Logarithms A logarithm is the inverse function for an exponent therefore we will review exponential functions first Review of Exponential Functions An exponential function has the general form where 0 b is called the base and x is called the exponent 1 or 1 1 aman a 2 am n amn3 ab m a b 4 am an am n a 6 0 5 a b m am bm b 6 0 6 am 1 am a 6 0 7 a1n n p a 8 a0 1 a 6 0 9 amn n p am n p a m where m and n are integers in properties 7 and 9 Logarithms De nition y logax if and only if x ay where a 0 In other words logarithms are exponents

Documentation Of Standard Typing Rules
https://blog.zulma.id/images/mineversal.png
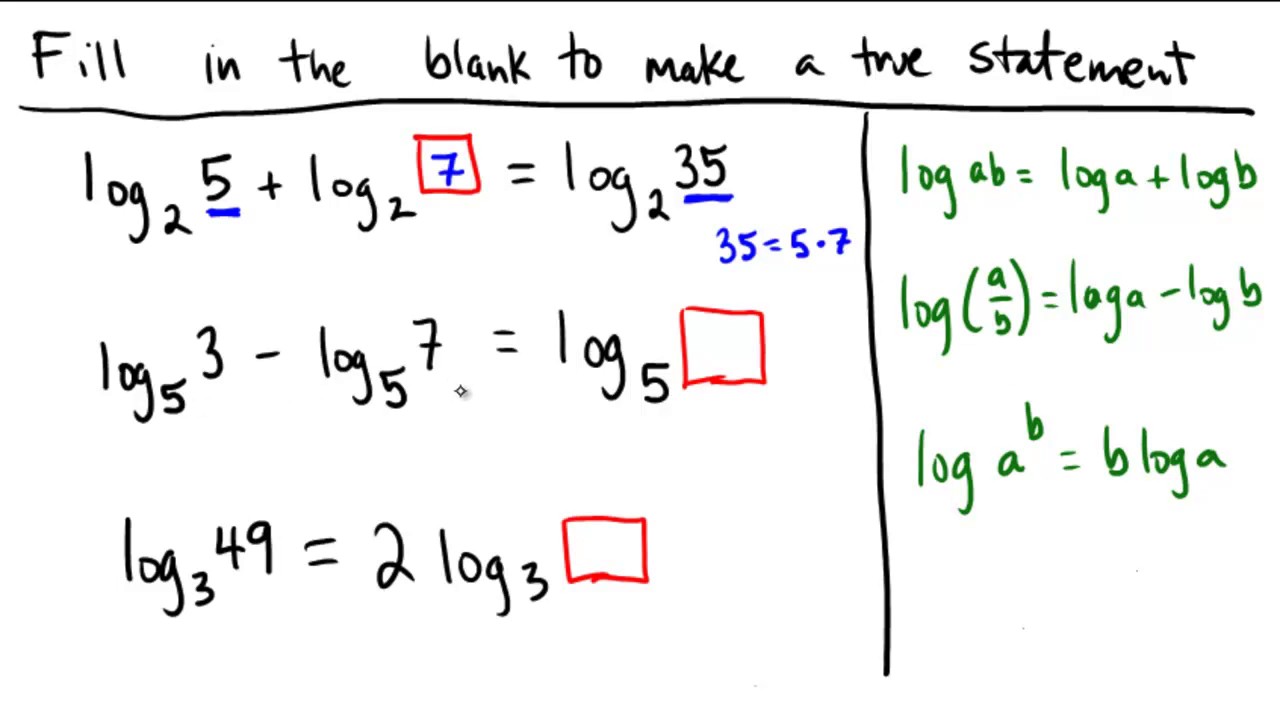
Logarithm Rules YouTube
https://i.ytimg.com/vi/CVpSjpaq4TI/maxresdefault.jpg
basic log rules pdf - Y 16 Now take it out of the exponential form and write it in logarithmic form Just like 23 8 converts to log 8 3 2 Ask your teacher about the last two examples They may show you a nice shortcut Finally we want to take a look at the Property of Equality for Logarithmic Functions Supp ose b 0 and b 1
