x 2 4x 1 0 complete the square Complete the square x 2 x 6 Description Complete the square for quadratic functions step by step complete the square calculator en Related Symbolab blog posts Middle School Math Solutions Polynomials Calculator Subtracting Polynomials In the previous post we talked about how to add polynomials In this post we will talk
As an example let s find the solution by completing the square for x2 0x 4 0 x 2 0 x 4 0 Eliminate the b term x2 4 0 x 2 4 0 Keep the x term on the left and move the constant to the right side by adding it to both sides x2 4 x 2 4 Solve by Completing the Square x 2 4x 1 x2 4x 1 x 2 4 x 1 To create a trinomial square on the left side of the equation find a value that is equal to the square of half of b b b 2 2 2 2 b 2 2 2 2 Add the term to each side of the equation x2 4x 2 2 1 2 2 x 2 4 x 2 2 1 2 2
x 2 4x 1 0 complete the square
x 2 4x 1 0 complete the square
https://qph.cf2.quoracdn.net/main-qimg-dffd971bed69cfdf1ad1aa52b5c67871-lq

X2 2x 8 0 Solve Given Equation By Completing Square Method YouTube
https://i.ytimg.com/vi/8NTfqVxDfxE/maxresdefault.jpg

Solving The Equation X 2 4x 7 0 By Completing The Square
https://i.pinimg.com/originals/98/1b/d1/981bd1d58182767a87bb45a7c1f4358e.jpg
Recall the short multiplication formula p q p 2pq q and note that we may apply it backwards to the left hand side of our equation with p x and q 3 We obtain x 3 16 Take the calculate the square root x 3 4 or x 3 4 which gives x 1 and x 7 Solve by Completing the Square 4x 2 4x 1 0 4x2 4x 1 0 4 x 2 4 x 1 0 Add 1 1 to both sides of the equation 4x2 4x 1 4 x 2 4 x 1 Divide each term in 4x2 4x 1 4 x 2 4 x 1 by 4 4 and simplify Tap for more steps x2 x 1 4 x 2 x 1 4
Example 2 x 2 4x 1 0 x 2 4x 1 adding 1 on both sides of the equation x 2 4x 4 1 4 Adding square of 2 on both sides of the equation x 2 4x 4 3 x 2 2 3 x 2 pm sqrt 3 x sqrt 3 2 or x sqrt 3 2 are the two solution to the quadratic equation above Certainly solving the above problems manually can be time consuming Step by Step Solution Reformatting the input Changes made to your input should not affect the solution 1 x2 was replaced by x 2 Step by step solution Step 1 Trying to factor by splitting the middle term 1 1 Factoring x2 4x 1 The first term is x2 its coefficient is 1 The middle term is 4x its coefficient is 4
More picture related to x 2 4x 1 0 complete the square
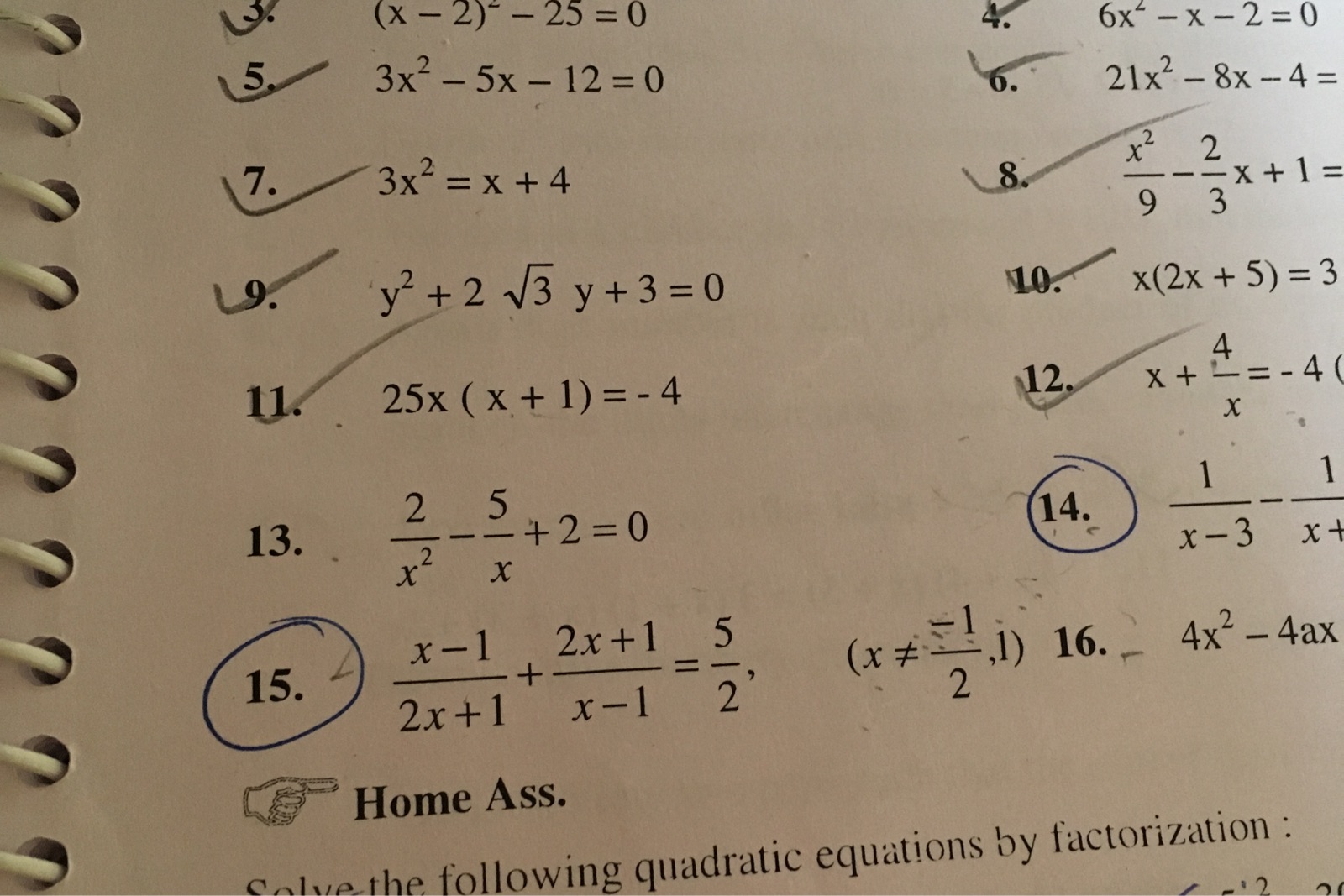
If X 2 2x 3 3x 4 2x 3 3x 4 4x 5 3x 5 5x 8 10x 17
https://dwes9vv9u0550.cloudfront.net/images/4478369/1d980e59-359c-40ef-8817-dc1a1db4e0f0.jpg

Solve The Quadratic Equation 3x 2 4x 1 0 By Completing The Square
https://i.ytimg.com/vi/8IO9z4lM8t4/maxresdefault.jpg

Solve By Completing The Square For X 2 2x 4 0 YouTube
https://i.ytimg.com/vi/w62q3CA3-YU/maxresdefault.jpg
Meave60 Jul 10 2015 x 2 5 2 5 Explanation x2 4x 1 0 Add 1 to both sides of the equation x2 4x 1 Force a perfect square trinomial on the left side by dividing the coefficient of the x term and squaring the result Add it to both sides of the equation 4 2 2 22 4 x2 4x 4 1 4 x2 4x 4 5 1 Divide the entire equation by 5 x 2 2x 23 5 2 Complete the square 2 2 1 1 2 1 Add 1 to both sides x 2 2x 1 23 5 1 3 Rewrite the left side as a binomial squared and add the fractions on the right x 1 2 28 5 4 Take square root of both sides sqrt x 1 2 sqrt 28 5 5 Simplify both radicals x 1
To complete the square first you want to get the constant c on one side of the equation and the variable s on the other side To do this you will subtract 8 from both sides to get 3x 2 6x 15 Next you want to get rid of the coefficient before x 2 a because it won t always be a perfect square Solve By Factoring Example 3x 2 2x 1 0 Complete The Square Example 3x 2 2x 1 0 After you click the example change the Method to Solve By Completing the Square Take the Square Root Example 2x 2 18 Quadratic Formula Example 4x 2 2x 1 0 About quadratic equations
How To Solve X 2 4x 1 0 By Completing The Square Quora
https://qph.cf2.quoracdn.net/main-qimg-93644b8c6e82aba59356dc574bb80ab9-lq

Solving The Equation X 2 4x 7 0 By Completing The Square YouTube
https://i.ytimg.com/vi/pPwj4Lqm52Q/maxresdefault.jpg
x 2 4x 1 0 complete the square - Step 1 Write the quadratic equation as x 2 bx c Coefficient of x 2 needs to be 1 If not take it as the common factor Step 2 Determine half of the coefficient of x Step 3 Take the square of the number obtained in step 1 Step 4 Add and subtract the square obtained in step 2 to the x 2 term