what is the slope of a horizontal line

what is the slope of a horizontal line How does a positive slope differ from a negative slope How does change in the slope affect the steepness of a line How do you determine the slope of 3 5 and 2 9

The slope of a horizontal line is 0 On a horizontal line all points have the same y value so the change in y over the change in x the rise over the run is always 0 over that change in x If we pick two different points on the line they must have different x values so we get 0 over a non 0 number which is 0 Since polar coordinates are defined by the radius and angle from the x axis horizontal and vertical tangent lines are found differently To find horizontal tangent lines set frac dy d theta 0 and to find vertical tangent lines set frac dx d theta 0

what is the slope of a horizontal line

what is the slope of a horizontal line
https://ds055uzetaobb.cloudfront.net/brioche/uploads/bdczPGKP4G-slopes.svg?width=1020

Como Calcular El Slope Design Talk
https://www.wikihow.com/images_en/thumb/4/45/Find-the-Slope-of-a-Line-Step-9-Version-3.jpg/v4-1200px-Find-the-Slope-of-a-Line-Step-9-Version-3.jpg

What Does A Horizontal Line Mean In Slope What Does
https://d20khd7ddkh5ls.cloudfront.net/horizontal_lines.jpg

For a straight line when expressed in the slope intercept form as y mx c we know that m is the slope of the line which it makes with x axis and c is intercept on the y axis Also that by definition m tan theta where theta is the angle line makes with the x axis theta tan 1m For the given problem m 5 This implies that the angle theta is more than 90 and lies in the The slope of the tangent line is the instantaneous slope of the curve So if we increase the value of the argument of a function by an infinitesimal amount then the resulting change in the value of the function divided by the infinitesimal will give the slope modulo taking the standard part by discarding any remaining infinitesimals

The slope of a horizontal tangent line is 0 To calculate the slope of a straight line we take a difference in the y dimension and divide it by the change in the x dimension of two points on the line slope y 1 y 2 x 1 x 2 assuming points x 1 y 1 and x 2 y 2 lie on the line For a horizontal line y 1 y 2 0 so Smallest tangent slope is at x 0 and will be 2 The first derivative is given by f x 5x 4 2 Recall that horizontal tangents will occur when f x 0 0 5x 4 2 2 5 x 4 x root 4 2 5 This is undefined therefore the function has no horizontal tangents To find the tangent with the minimum slope we seek to minimize 5x 4 2 You re right that the derivative

More picture related to what is the slope of a horizontal line

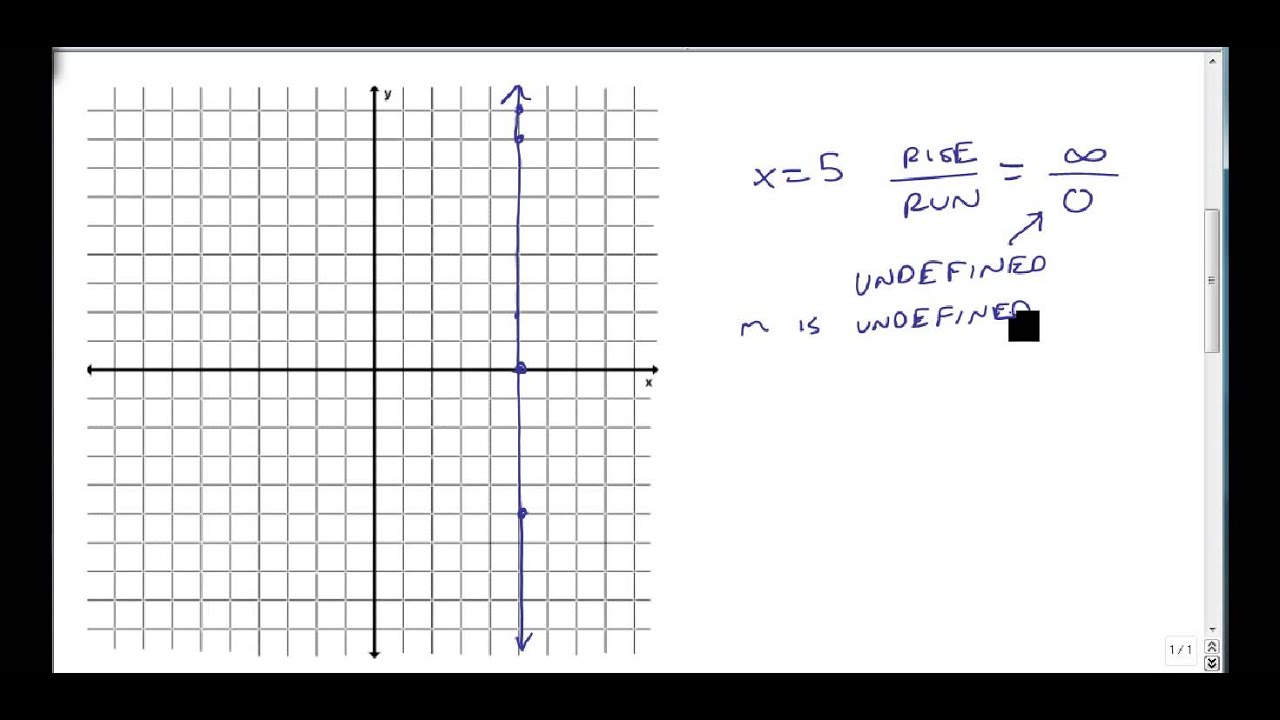
Find The Slope Of Vertical And Horizontal Lines YouTube
https://i.ytimg.com/vi/Pfj8lkS6N50/maxresdefault.jpg

The Slope Of A Horizontal Line Is Zero
https://www.thoughtco.com/thmb/buZIZoiwe6goBFfSKdo4B3u_V9Y=/5400x3259/filters:fill(auto,1)/man-pushing-snail-up-slowly-ascending-line-on-graph-145071961-5a1dc49422fa3a003778124c.jpg

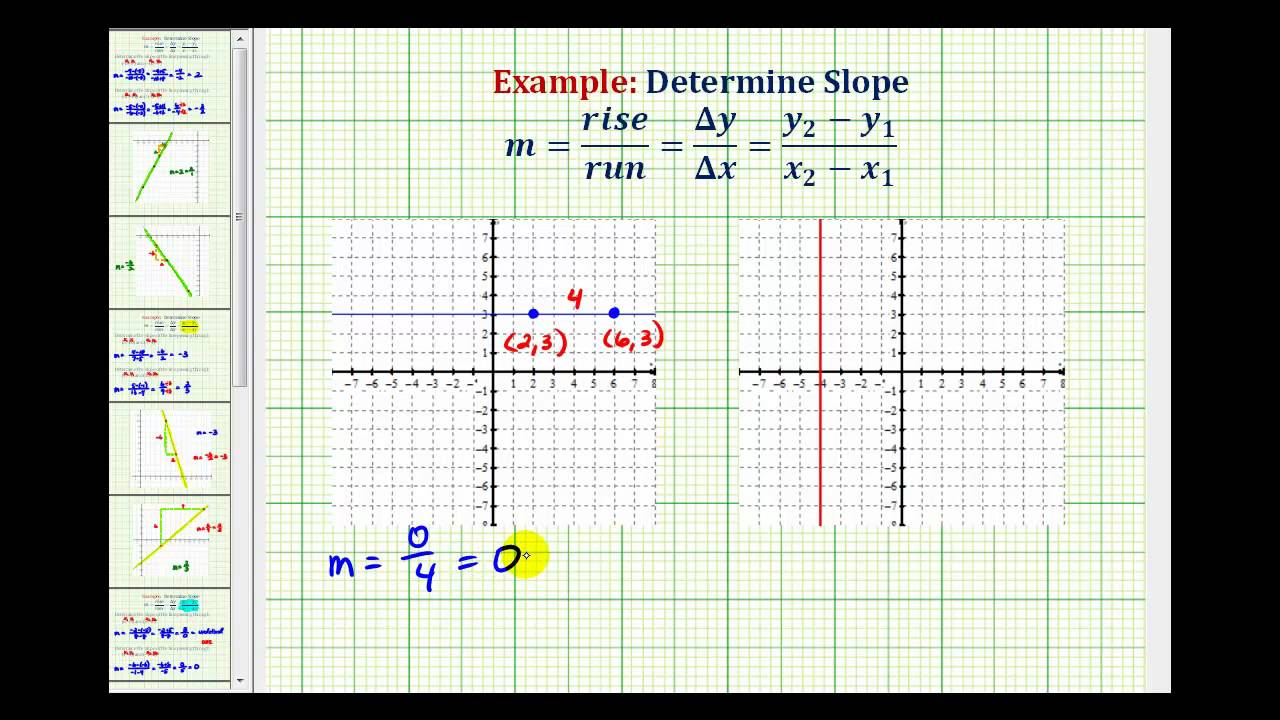
Ex Determine The Slope Given The Graph Of A Horizontal And Vertical
https://i.ytimg.com/vi/dJuFWXn7zJM/maxresdefault.jpg

The resulting point slope form of the equation can be rewritten into slope intercept form which in this case is y 7 8x 11 2 Explanation To write the equation of a line that passes through two points you first need to find the slope m of the line The slope is calculated using the formula m y2 y1 x2 x1 Using the provided Any horizontal line parallel to the x axis has a zero slope The horizontal line passing through 2 3 has equation y 3 as it passes through all points in the plane with a y coordinate of 3 as may be seen in the graph graph y 0 001x 3 0 10 10 5 5

[desc-10] [desc-11]

Slope Of A Line YouTube
https://i.ytimg.com/vi/8YF1EUP1nmI/maxresdefault.jpg

What Is The Slope Of A Straight Line
https://www.matematica.pt/en/images/faq/slope.png

what is the slope of a horizontal line - The slope of a horizontal tangent line is 0 To calculate the slope of a straight line we take a difference in the y dimension and divide it by the change in the x dimension of two points on the line slope y 1 y 2 x 1 x 2 assuming points x 1 y 1 and x 2 y 2 lie on the line For a horizontal line y 1 y 2 0 so