what is the integral of a derivative Since int a xf t mathrm dt f x f a tag 1 the short answer is that the integral of the derivative is the original function up to a constant Of course 1 isn t true without restrictions But if f is continuous then yes 1 holds
Integration derivatives leibniz integral rule Share Cite Follow edited Nov 14 2020 at 7 32 Martin Sleziak 53 9k 20 195 367 asked May 1 2012 at 2 37 Jiew Meng 4 603 22 71 100 7 f x dx is a The derivative of that is zero For the f x you have you need the fundamental theorem of calculus and the chain rule Find the derivative of an integral d d x 0 x t 5 d t To find the derivative apply the second fundamental theorem of calculus which states that if f is continuous on a b and a x b the derivative of an integral of f can be calculated d d x a x f t d t f x x 5
what is the integral of a derivative
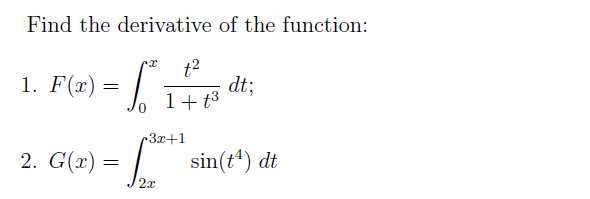
what is the integral of a derivative
https://d2vlcm61l7u1fs.cloudfront.net/media/558/558964cb-b10e-4482-9a78-c3258c555e9f/phpNvViqM.png

Derivative And Integral Formula Wallpaper 2 By SawyerTHEBEST On DeviantArt
https://images-wixmp-ed30a86b8c4ca887773594c2.wixmp.com/i/2bbff7ca-f553-46c0-b08b-0504dfc9b328/d5yfd6f-d3e915e9-2ccd-446a-9370-4b65f070c17f.png
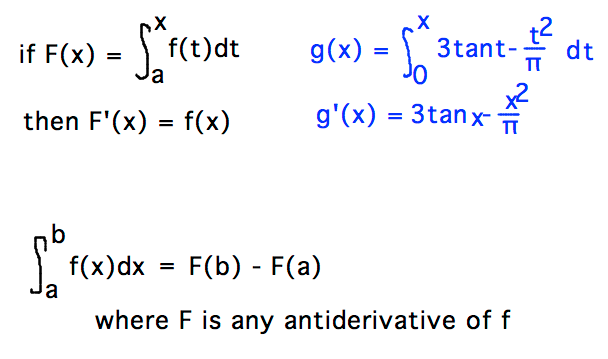
Geneseo Math 221 10 Definite Integral Intro
https://www.geneseo.edu/~baldwin/math221/fall2014/10/fundamental.png
The Fundamental Theorem of Calculus Part 1 shows the relationship between the derivative and the integral The Fundamental Theorem of Calculus Part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand The derivative of x is 1 This shows that integrals and derivatives are opposites Now For An Increasing Flow Rate Imagine the flow starts at 0 and gradually increases maybe a motor is slowly opening the tap As the flow rate increases the tank fills up faster and faster Integration With a flow rate of 2x the tank volume increases by x 2
Dx when x approaches zero gives the actual derivative and integral Note this is a computer model and actually uses a very small x to simulate dx and can make erors For true derivatives refer to Derivative Rules and for integrals refer to Similar to how one can think of a derivative as a function that yields a tangent slope for any given x x one can create a function using a definite integral that gives the area under the graph of some non negative valued function from some specified value to any given x x
More picture related to what is the integral of a derivative

Learn How To Find The Derivative Of The Integral YouTube
https://i.ytimg.com/vi/hXLu_Q47NYw/maxresdefault.jpg

Integral Versus Derivative Poster By Qqqueiru Redbubble
https://ih1.redbubble.net/image.433764046.8100/flat,550x550,075,f.u8.jpg

Question Video Finding The Derivative Of A Function Defined By An
https://media.nagwa.com/874186171812/en/thumbnail_l.jpeg
The basic idea of Integral calculus is finding the area under a curve To find it exactly we can divide the area into infinite rectangles of infinitely small width and sum their areas calculus is great for working with infinite things Integral calculus gives us the tools to answer these questions and many more Surprisingly these questions are related to the derivative and in some sense the answer to each one is the opposite of the derivative
[desc-10] [desc-11]

Question Video Finding The Derivative Of A Function Defined By An
https://media.nagwa.com/785198728368/en/thumbnail_l.jpeg

Question Video Finding The Second Derivative Of Rational Functions Nagwa
https://media.nagwa.com/902150342140/en/thumbnail_l.jpeg
what is the integral of a derivative - The Fundamental Theorem of Calculus Part 1 shows the relationship between the derivative and the integral The Fundamental Theorem of Calculus Part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand