what does a system of linear equations with infinite solutions look like If a pair of the linear equations have unique or infinite solutions then the system of equation is said to be a consistent pair of linear equations Thus suppose we have two equations in two variables as follows a 1 x b 1 y c 1 1 a 2 x b 2 y c 2 2
A system of linear equations has infinite solutions when the graphs are the exact same line Want to learn more about the number of solutions to systems of equations Check out this video Example system with one solution We re asked to find the number of solutions to this system of equations y 6 x 8 3 x y 4 Consider the following linear system x y 0 x y 0 There are obviously infinite solutions to this system as long as x y x y we have a solution We can picture all of these solutions by thinking of the graph of the equation y x y x on the traditional x y x y coordinate plane
what does a system of linear equations with infinite solutions look like

what does a system of linear equations with infinite solutions look like
https://media.nagwa.com/384163156847/en/thumbnail_l.jpeg
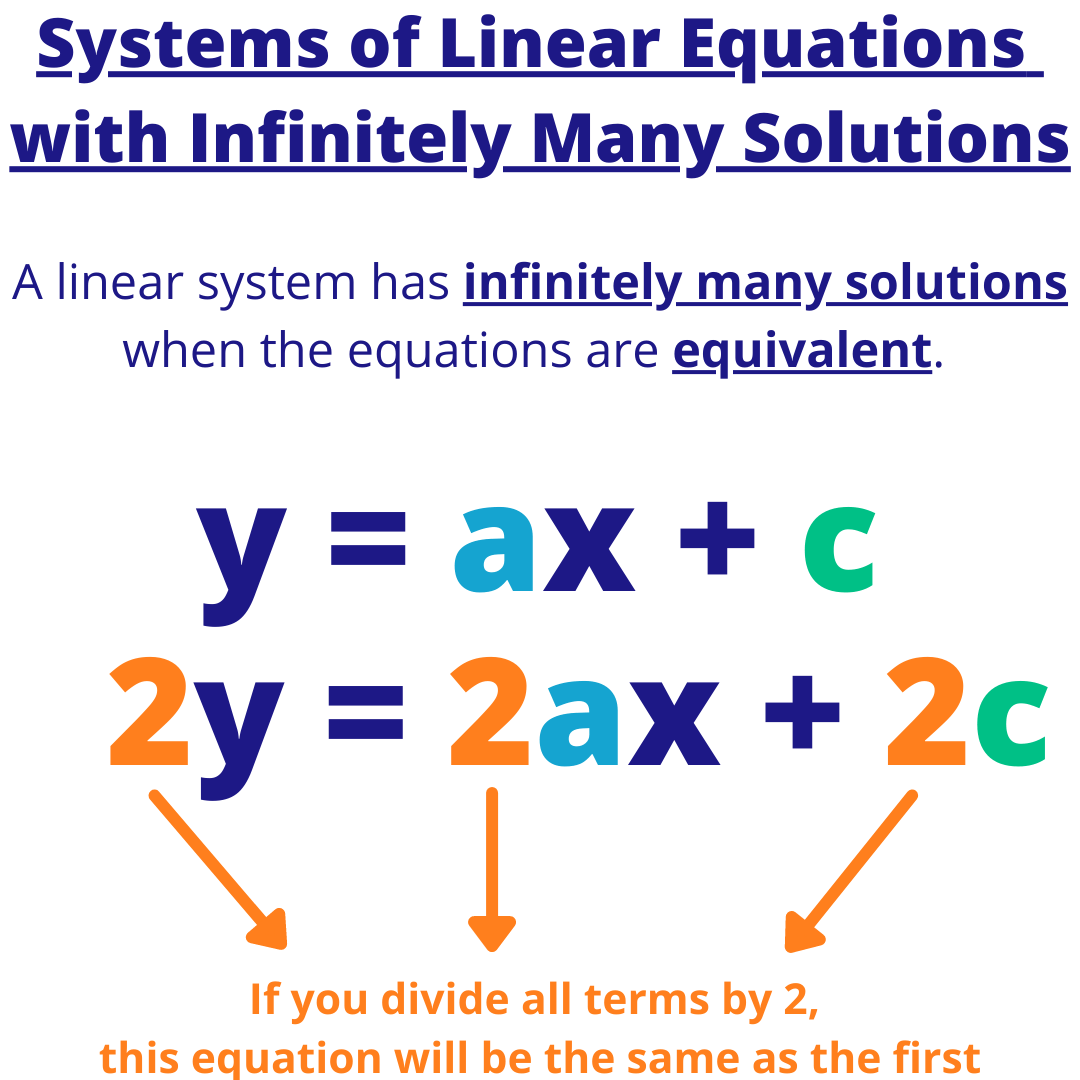
Systems Of Linear Equations With Infinite Solutions Examples Expii
https://d20khd7ddkh5ls.cloudfront.net/systems_of_linear_equations_with_infinitely_many_solutions.png
Simple Ways To Solve Equations With Infinite Solutions
https://dailybulletin.com.au/images/00/types.jpg
Let s use the second equation and the variable y it looks the simplest equation Write one of the equations so it is in the style variable We can subtract x from both sides of x y 8 to get y 8 x Now our equations look like this 3x 2y 19 y 8 x Now replace y with 8 x in the other equation 3x 2 8 A linear system with infinite solutions has 2 equations that end up being the same line You could change your equation into slope intercept form It would be the same equation but look different Another way you could multiply or divide your equation by a constant and you have an equivalent equation
A consistent pair of linear equations will always have unique or infinite solutions Example 1 Here are two equations in two variables a1x b1y c1 1 a2x b2y c2 2 If a1 a2 b1 b2 c1 c2 Then the equation is a consistent and dependent equation that has infinitely many solutions Let s look at a system of equations that has infinite solutions 22x 11y 4414x 7y 28 Try simplifying the top equation What do you notice 2x y 42x y 4 These are same equation They ll both be exactly the same line on a graph In other words they ll overlap completely This is easier to tell because both equations are in standard form
More picture related to what does a system of linear equations with infinite solutions look like
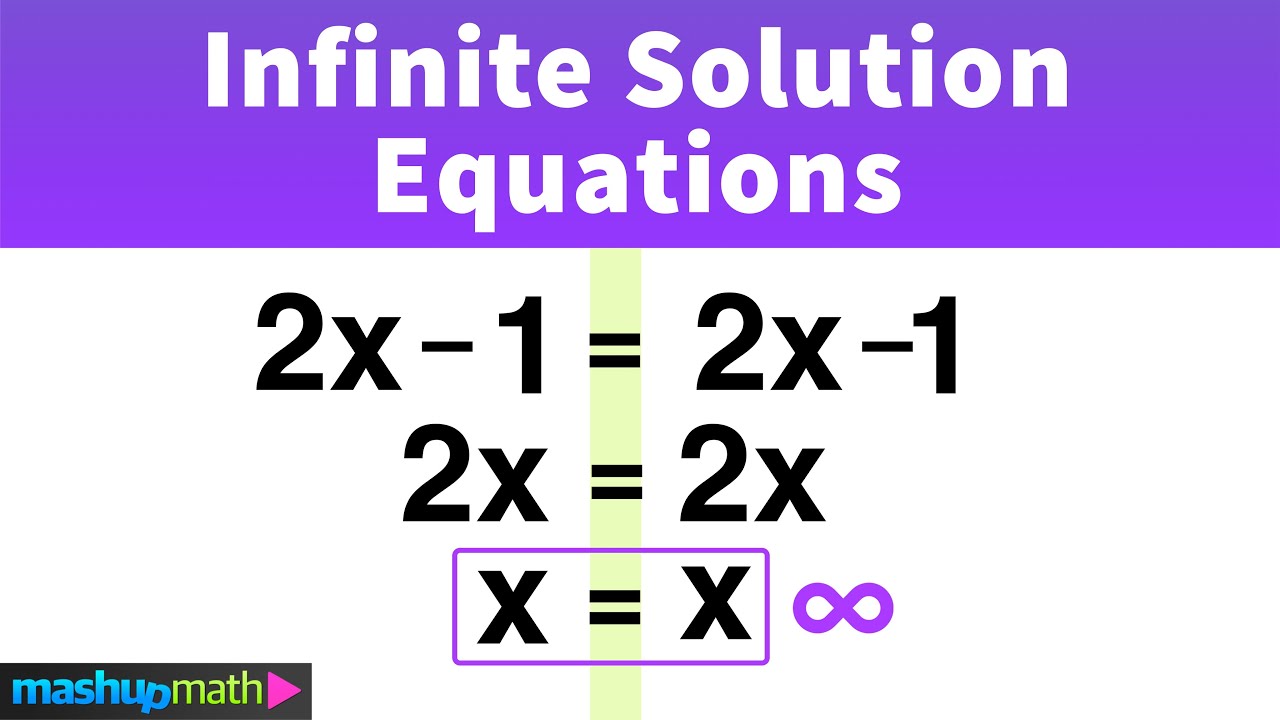
Infinite Solutions Equations Explained YouTube
https://i.ytimg.com/vi/o_zob5jIn6E/maxresdefault.jpg

In This Video Explore Linear Equations In One Variable With One
https://i.pinimg.com/736x/38/13/1c/38131cad0c7f438ba92a9ce09015ff0f.jpg

Linear Systems With Infinite Solutions Example 2 Video Algebra
https://s3.amazonaws.com/ck12bg.ck12.org/curriculum/105533/thumb_540_50.jpg
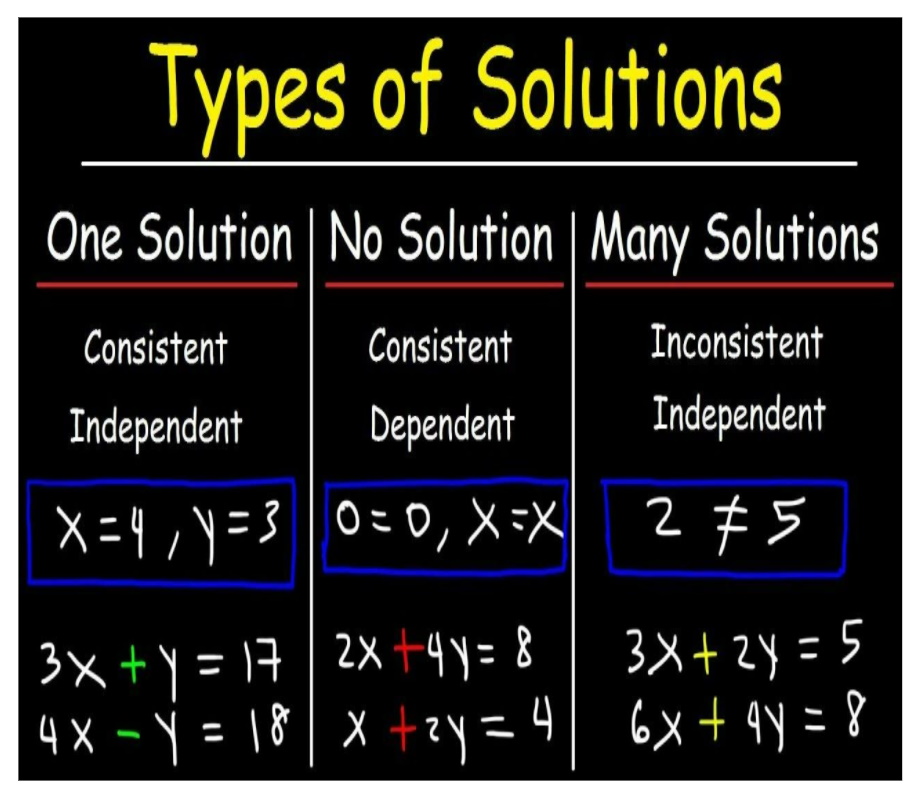
In a system of equations the solution is the point where the graphed lines intersect Parallel lines never intersect so they have zero solutions Lines that are the same are always together so those are the ones with infinite solutions Systems that have an infinite number of solutions are those which after elimination result in an expression that is always true such as 0 0 0 0 Graphically an infinite number of solutions represents a line or coincident plane that serves as the intersection of three planes in space
Case III Infinite Solutions This is the rarest case and only occurs when you have the same line Consider for instance the two lines below y 2x 1 and 2y 4x 2 These two equations are really the same line Example of a system that has infinite solutions Infinite Solutions Sometimes the two equations will graph as the same line in which case we have an infinite number of solutions No Solution When the lines that make up a system are parallel there are no solutions because the two lines share no points in common

Solve The System Of Equations X Y 10 And Y 4x Using The
https://i.ytimg.com/vi/kc6stXQ6I3Y/maxresdefault.jpg

Linear System Of Equations With Infinitely Many Solutions YouTube
http://i.ytimg.com/vi/tquUDsduwys/maxresdefault.jpg
what does a system of linear equations with infinite solutions look like - A consistent pair of linear equations will always have unique or infinite solutions Example 1 Here are two equations in two variables a1x b1y c1 1 a2x b2y c2 2 If a1 a2 b1 b2 c1 c2 Then the equation is a consistent and dependent equation that has infinitely many solutions