on the square roots of triangular numbers It is well known that the balancing numbers are the square roots of the triangular numbers and are the solutions of the Diophantine equation 1 2 n 1 n
100 MAY ON THE SQUARE ROOTS OF TRIANGULAR NUMBERS c B2n B2n B2n v d B 2n l B n Bn l Bn l Proof From 9 it follows that Bn l Write Nk for the kth square triangular number and write sk and tk for the sides of the corresponding square and triangle so that Define the triangular root of a triangular number N n n 1 2 to be n From this definition and the quadratic formula Therefore N is triangular n is an integer if and only if 8N 1 is square Consequently a square
on the square roots of triangular numbers
on the square roots of triangular numbers
https://thirdspacelearning.com/wp-content/uploads/2022/05/Triangular-numbers-featured-card.png

My Math Resources Squares Square Roots Poster
https://i0.wp.com/mymathresources.com/wp-content/uploads/2019/12/IMG_9231.jpg?fit=1200%2C1200&ssl=1
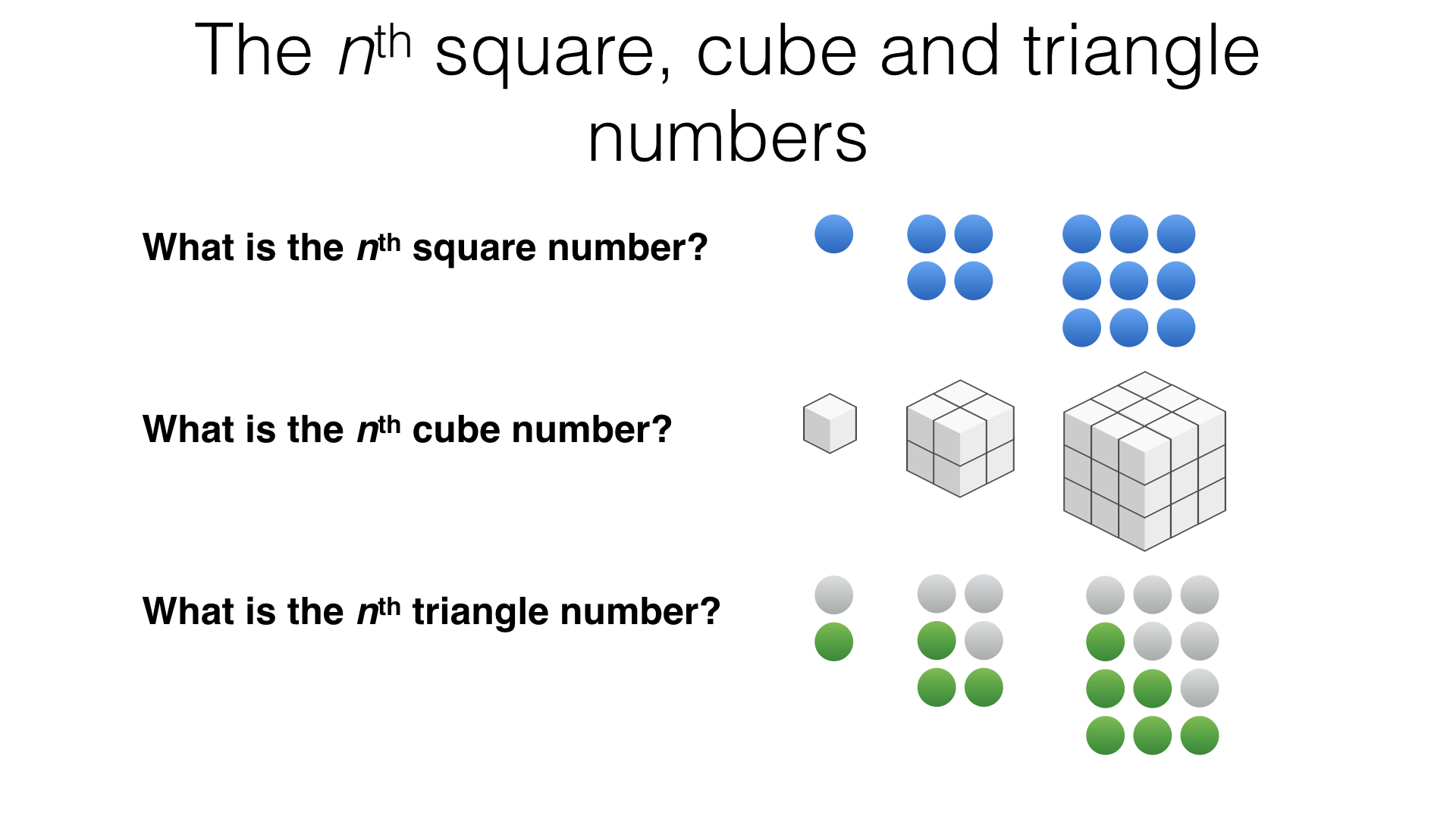
A24a Recognising And Using Square Cube And Triangular Numbers
https://bossmaths.com/wp-content/uploads/A24as.010.png
By analogy with the square root of x one can define the positive triangular root of x as the number n such that Tn x which follows immediately from the quadratic formula So an integer x is triangular if and only if 8x 1 is a square Equivalently if the positive triangular root n of x is an integer then x is the nth triangular number In the joint paper On the square roots of triangular numbers published in The Fibonacci Quarterly in 1999 Behera and Panda introduced balancing numbers and studied many important
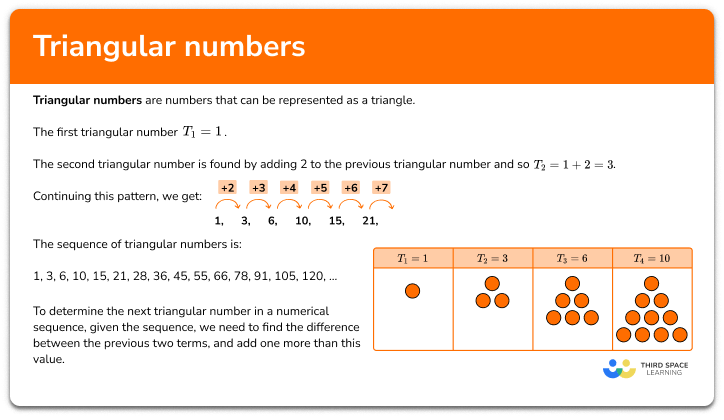
Triangular numbers are numbers that can be represented as a triangle The numbers form a sequence known as the triangular numbers The first triangular number T 1 1 T 1 1 The second triangular Formula The formula to find the n th triangular number is T n i 1 n i n n 1 2 Derivation As we know the sum of the first n natural numbers can be
More picture related to on the square roots of triangular numbers

How To Take Square Roots Of 45 Brainly in
https://hi-static.z-dn.net/files/d78/0367833905f5e82fb50a50cf020a3a7c.png

Find The Square Roots Of The Followingnumbers By The Long Division
https://hi-static.z-dn.net/files/d52/22d52873798ba2088883e2edc29f5c37.jpg
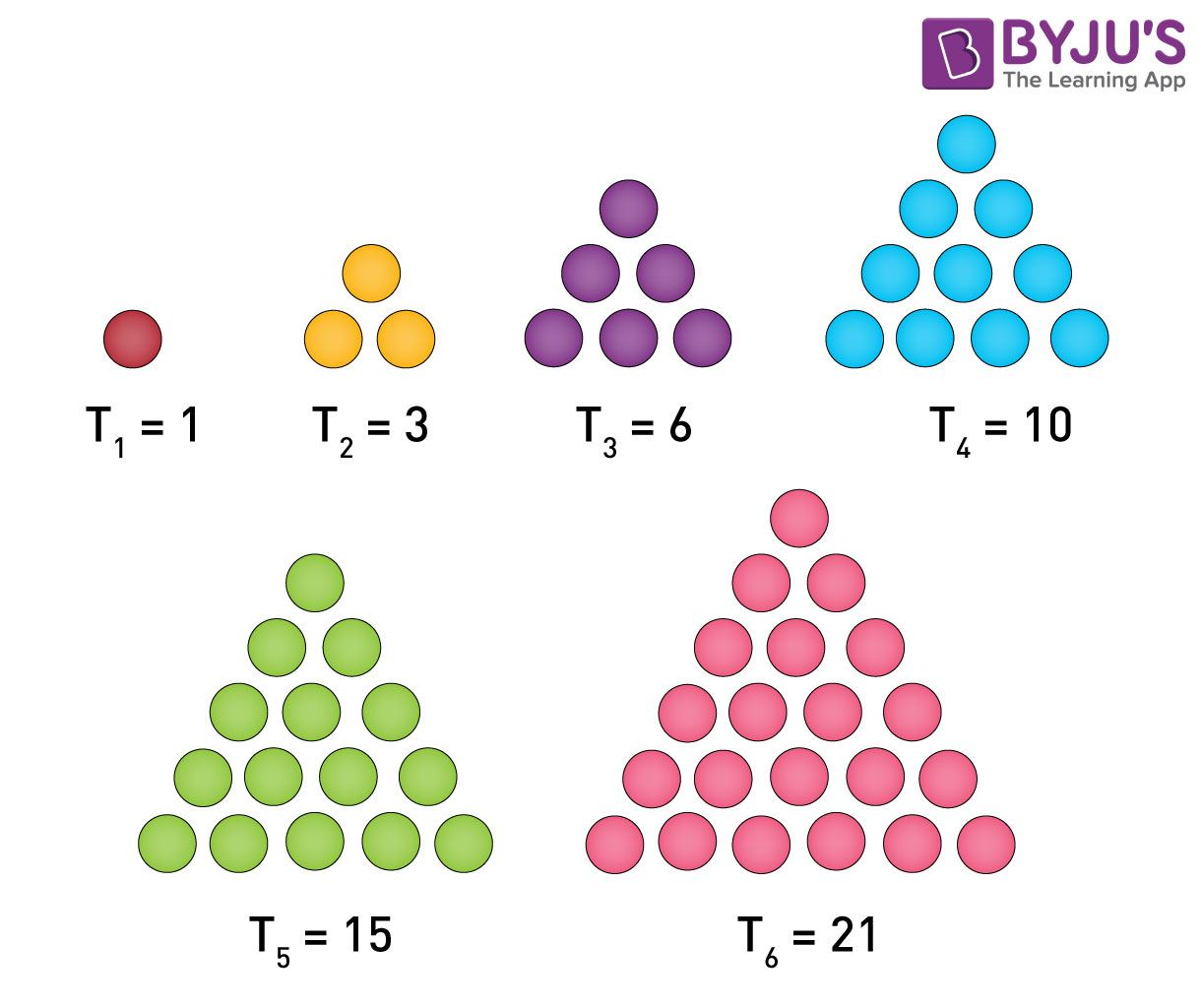
Sequences Definition Rules And Examples Of Sequences
https://cdn1.byjus.com/wp-content/uploads/2021/07/triangular-numbers-sequence.png
Greetings from The On Line Encyclopedia of Integer Sequences A001109 a n 2 is a triangular number a n 6 a n 1 a n 2 with a 0 0 a 1 1 Formerly M4217 Answer 20 comments 58 votes Upvote Downvote Flag more Aerin 4 years ago Square root of 4 is 2 An easier way to solve the square root for small and
Proof S ince x is a balancing num ber 8x2 1 is a perfect square and 8x2 8x2 l 4 x 2 8 x 2 1 is a triangular num ber w hich is also a perfect square therefore its An arrangement of triangular numbers starting with the 0th triangular number is as follows Numbers 0 through 1 3 6 10 15 21 28 36 45 55 66 78 91

Using The Long Division Method Find The Square Roots Of The 390625
https://hi-static.z-dn.net/files/de9/94cea3bb1a82f9137d5a5e9038b587af.jpg

Find The Square Roots Of The Following Numbers By Division Method 3600
https://hi-static.z-dn.net/files/db6/0f4b652541b90660fbd61ce168126294.jpg
on the square roots of triangular numbers - In the joint paper On the square roots of triangular numbers published in The Fibonacci Quarterly in 1999 Behera and Panda introduced balancing numbers and studied many important