logarithm properties proof pdf Use the exponent rules to prove logarithmic properties like Product Property Quotient Property and Power Property Learn the justification of these properties with ease
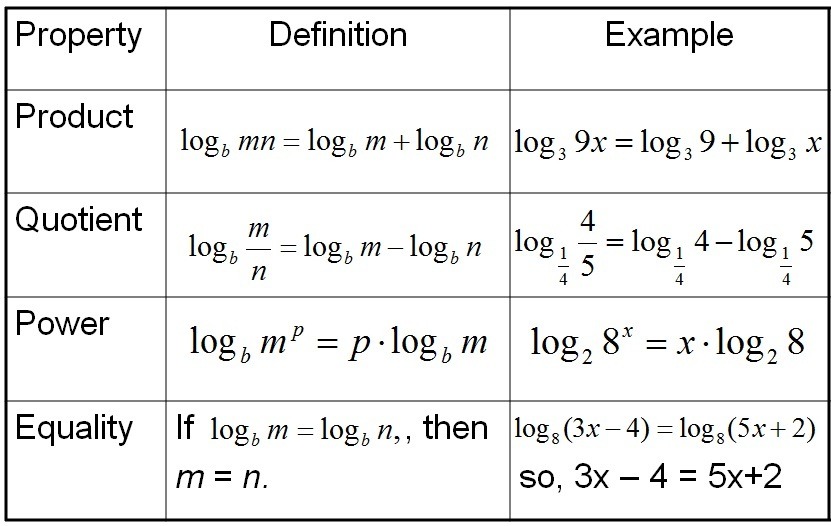
We are going to introduce the 4 most important rules of logarithms upon which all other rules can be derives Here is a summary of them we will go through each one including examples and brief proofs Logarithm Properties log b a log b c log b a c log Key Takeaways Given any base b 0 and b 1 we can say that logb1 0 logbb 1 log1 bb 1 and that logb 1 b 1 The inverse properties of the logarithm are logbbx x and blogbx x where x 0 The product property of the logarithm allows us to write a product as a sum logb xy logbx logby
logarithm properties proof pdf
logarithm properties proof pdf
https://www.geogebra.org/resource/c2Wm7GgS/Akp8l8lD1Uv0tp37/material-c2Wm7GgS.png

Proofs Of Logarithm Properties video Lessons Examples And Solutions
https://i.pinimg.com/originals/bb/c9/ba/bbc9ba74d4d3d0cba57488959559b69d.png

Exponent Power Rule Worksheet Worksheetfun Log Rules Learning
https://i.pinimg.com/originals/67/3e/9e/673e9ebff1ebf38e84e9aaec6717d804.gif
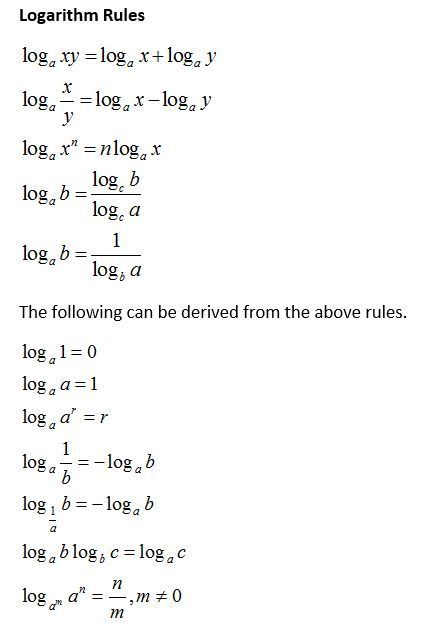
Proof Set b loga xy c loga x d loga y so that we have ab xy ac x ad y We then get ab xy ab acad ab ac d b c d loga xy loga x loga y Theorem 2 4 loga x y loga x loga y Proof Set b loga x y c loga x d loga y so that ab x y ac x ad y We then get Solve Solution 1 n n 0 we know 1 273 9 8 which satisfies x Using the propeO clw n Therefore x 8 Laws of Logarithms Logarithm of a Power Power Law logc n loge wherec 0 c 1 x 0 This is the logarithmic form of the exponent law cm cmn Proof Let logc x m
17 Properties of Logarithms Learning Objectives Use the definition of common and natural logarithms in solving equations and simplifying expressions Use the change of base property to evaluate logarithms Solve exponential equations using logarithmic properties Combine and or expand logarithmic expressions Section 5 3 Properties of Logarithms Objective 1 Using the Product Rule Quotient Rule and Power Rule for Logarithms Let b 0 b 1 u and v represent positive numbers and r be any real number The Product Rule for Logarithms is log b uv log b u log b v The Quotient Rule for Logarithms islog u log b u log b v v
More picture related to logarithm properties proof pdf

Logarithm Formulas symbol symbols mathematics maths
https://i.pinimg.com/originals/13/88/b8/1388b8d6f8530a4519efe9a73f0ec2c4.png
How To Apply The Logarithm Rules Product Rule Quotient Rule Power
https://i.pinimg.com/736x/4a/f7/5f/4af75f7706105dfa727fbe8d2aad98de.jpg

A Proof Of The Logarithm Properties YouTube
http://i.ytimg.com/vi/_ZFe9Z0lzQE/maxresdefault.jpg
Proof of 1 Let u log b x and v log b y so bu x and bv y Thus xy bubv bu v so log b xy u v log b x log b y Proof of 2 Let u log b x and v log b y so bu x and bv y Thus x y bu bv bu v so log b x y u v log b x log b y Proof of 3 Let u log b xy so bu xy Also x blog b x so xy blog b x In this lesson we will prove three logarithm properties the product rule the quotient rule and the power rule Before we begin let s recall a useful fact that will help us along the way log b b c c In other words a logarithm in base b reverses the effect of a base b power Why is this true again log b a b a log b b c b b c c
Suppose we pick a number 8 say Suppose we find its logarithm to base 2 to evaluate log2 8 Suppose we now raise the base 2 to this power 2log2 8 Because 8 23 we can write this as 2log2 23 Using the laws of logarithms this equals 23log2 2 which equals 23 or 8 since log2 2 1 Properties of Logarithms Assume that is a constant greater than 1 Let C log B This logarithm function is by definition the inverse of the function C B The domain of C log B is ab The range is In the statements below assume that BC and are arbitrary positive numbers 1 log 2 log 3 If B log B

Logarithmic Equations Worksheet With Answers
https://static.docsity.com/documents_first_pages/2021/04/20/a45b41a51e4b0a71f6f6fbba867f6871.png
Proof Of Logarithm Power Change Mathematics Stack Exchange
http://i.stack.imgur.com/F51wy.jpg
logarithm properties proof pdf - The logarithm of 1 to any base is always 0 and the logarithm of a number to the same base is always 1 In particular log 10 10 1 and log e e 1 Exercises 1 Use the rst law to simplify the following a log 10 6 log 10 3 b logx logy c log4x logx d loga logb2 logc3 2 Use the second law to simplify the following a log 10 6