log rules math Learn the eight 8 log rules or laws to help you evaluate expand condense and solve logarithmic equations Try out the log rules practice problems for an even better understanding
Logarithm Rules and Properties There are certain rules based on which logarithmic operations can be performed The names of these rules are Product rule Division rule Power rule Exponential Rule Change of base rule Base switch rule Derivative of log Integral of log Let us have a look at each of these properties one by one Product Rule Log rules are rules that are used to operate logarithms Since logarithm is just the other way of writing an exponent we use the rules of exponents to derive the logarithm rules There are mainly 4 important log rules which are stated as follows product rule log b mn log b m log b n quotient rule log b m n log b m log b n power
log rules math
log rules math
https://imgv2-2-f.scribdassets.com/img/document/451343007/original/6ae4a12fb0/1607714239?v=1

Log Rules Narural Log Rules Rules Of Ln Logarithm Rules
https://d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/log-or-logarithm-rules-1647317216.png

Logarithm Rules aka Log Laws Explained With Examples Maths For Kids
https://i0.wp.com/maths.forkids.education/wp-content/uploads/2020/05/Log-Laws-rules-of-logarithm.jpg?fit=1000%2C798&ssl=1
Introduction to Logarithms In its simplest form a logarithm answers the question How many of one number multiply together to make another number Example How many 2 s multiply together to make 8 Answer 2 2 2 8 so we had to multiply 3 of the 2 s to get 8 So the logarithm is 3 How to Write it We write it like this log2 8 3 For simplicity we ll write the rules in terms of the natural logarithm ln x The rules apply for any logarithm log b x except that you have to replace any occurence of e with the new base b The natural log was defined by equations eqref naturalloga and eqref naturallogb
Log rules There are a number of logarithm rules properties and identities that can be used when working with logarithms They can be particularly useful for manipulating and solving algebraic expressions or equations Three basic logarithm rules are the product quotient and power rules In this lesson we will prove three logarithm properties the product rule the quotient rule and the power rule Before we begin let s recall a useful fact that will help us along the way log b b c c In other words a logarithm in base b reverses the effect of a base b power Why is this true again log b a b a log b b c b b c c
More picture related to log rules math

Logarithm Rules Study Pivot 2 Medium
https://cdn-images-1.medium.com/max/1600/1*AvjkLYUMrXaJNNMAmlotiw.png
Log Rules Yup Math
https://d33wubrfki0l68.cloudfront.net/4c93c5b12da8488fa19ba04718426a72b3377c97/19145/ef925d0850547459e95bbfced9fe7b36/log_rules.svg

Logarithm Rules video Lessons Examples And Solutions
https://www.onlinemathlearning.com/image-files/logarithm-rules.png
What is a logarithm Logarithms are another way of thinking about exponents For example we know that 2 raised to the 4 th power equals 16 This is expressed by the exponential equation 2 4 16 Now suppose someone asked us 2 raised to which power equals 16 The answer would be 4 This is expressed by the logarithmic equation log 2 Test your knowledge of the skills in this course Start Course challenge Math Algebra 2 Unit 8 Logarithms 900 possible mastery points Mastered Proficient Familiar Attempted Not started
[desc-10] [desc-11]

Rules Of Logarithms Exponents I Deal With Logarithms Everyday Sighh
https://s-media-cache-ak0.pinimg.com/originals/7b/b6/7e/7bb67e9d2a4bcff3dedfeb1e6b86982b.gif
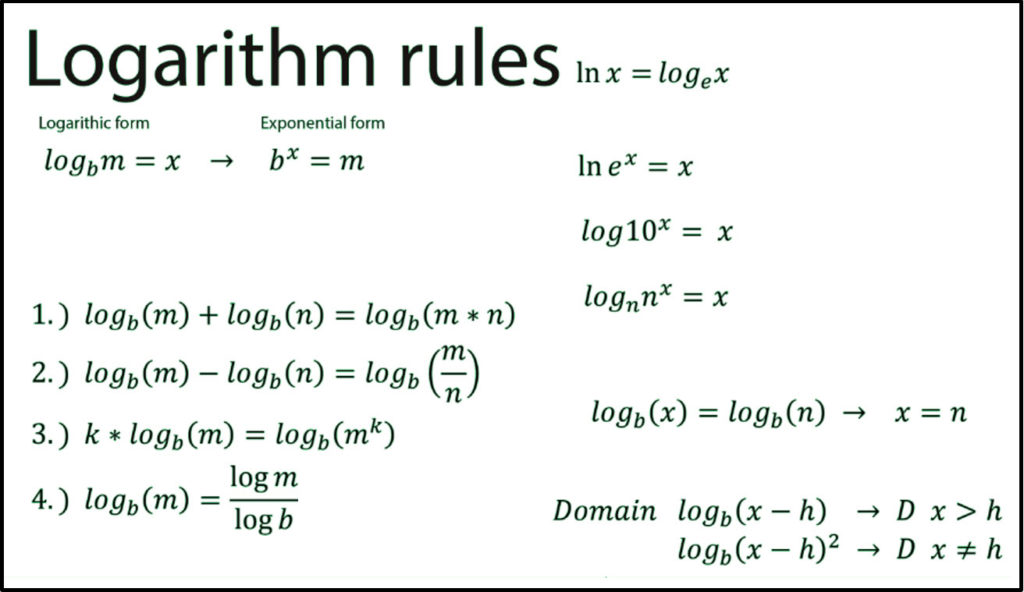
Logarithms
https://andymath.com/wp-content/uploads/2019/07/Lognotes-1024x592.jpg
log rules math - [desc-14]

