integral of product of two functions inequality First what does it mean to say that the integral of a product of two functions is less than a weighted sum of the squares of their individual 2 norms I think my confusion stems in large
If you restate that inequality with absolute values then it is true Consider the Riemann integral in terms of the limit of a series Let M sup f x x R and a Theorem 1 2 13 Inequalities for Integrals Let a le b be real numbers and let the functions f x and g x be integrable on the interval a le x le b text If f x ge 0
integral of product of two functions inequality

integral of product of two functions inequality
https://i.stack.imgur.com/shrNT.png

Integration By Parts Integral Of Xe x cos x Dx YouTube
https://i.ytimg.com/vi/hWwmgYrRE4Q/maxresdefault.jpg

Rules For Integrating Linear Combinations Of Functions YouTube
https://i.ytimg.com/vi/-QlWEG2j_mM/maxresdefault.jpg
In mathematical analysis H lder s inequality named after Otto H lder is a fundamental inequality between integrals and an indispensable tool for the study of L spaces The numbers p and q above are said to be H lder conjugates of each other The special case p q 2 gives a form of the Cauchy Schwarz inequality H lder s inequality holds even if fg 1 is infinite the right hand side also being infinite in that case Conversely if f is in L and g is in L th Properties of the Riemann Integral Let f be a bounded real valued function on a b If U f P and L f P denote upper and lower sums for a given partition P of a b then we say that f is
Let f x and g x be continuous functions de ned on a b where f x g x for all x in a b The area of the region bounded by the curves y f x y g x and the lines x a and b is b f x The Product Rule enables you to integrate the product of two functions For example through a series of mathematical somersaults you can turn the following equation
More picture related to integral of product of two functions inequality

Integration By Parts Wize University Calculus 1 Textbook Wizeprep
https://d3rw207pwvlq3a.cloudfront.net/attachments/000/143/910/images/img_poster.0000000.jpg?1615772245
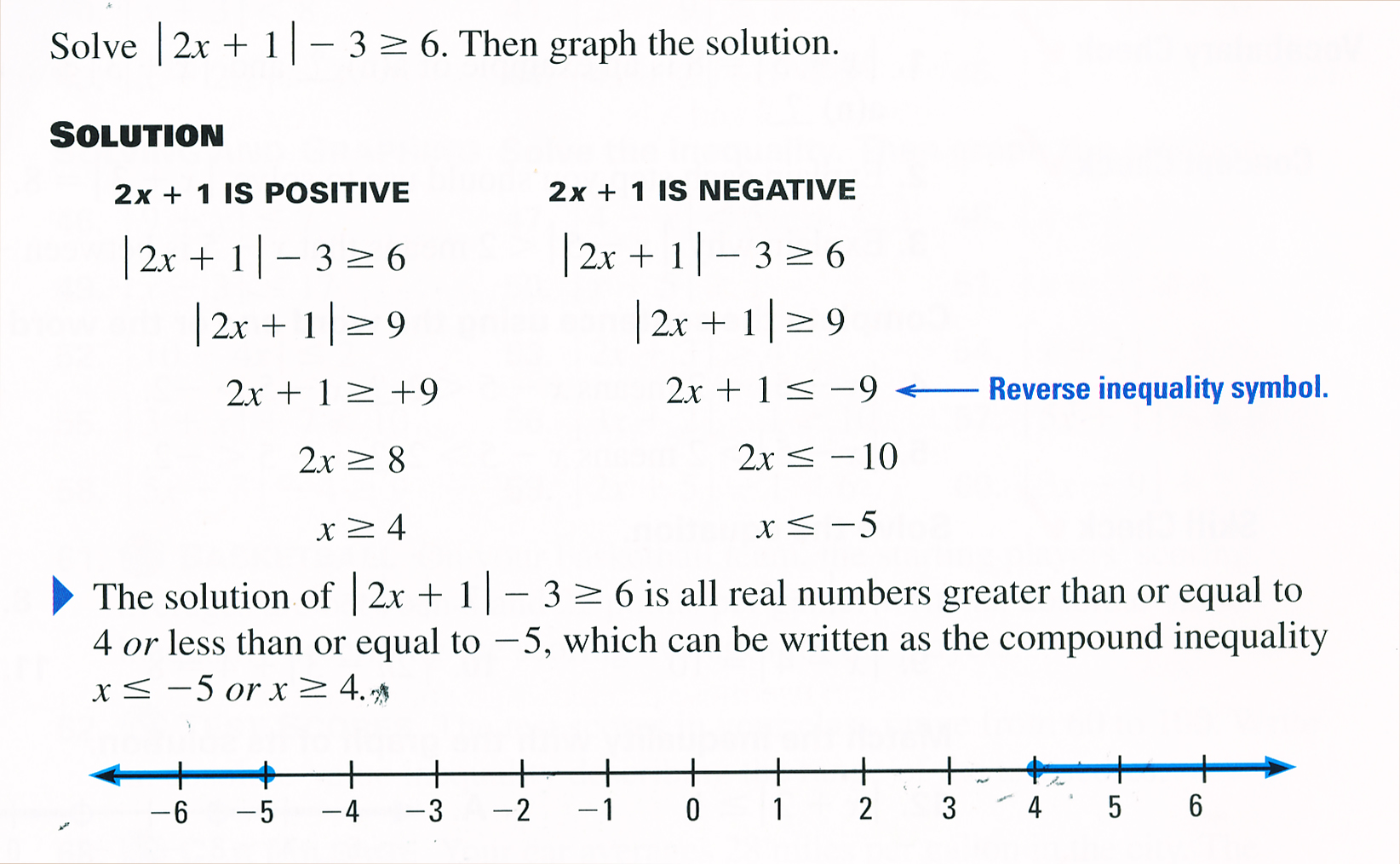
Absolute Value Equations And Inequalities INTEGRAL SK
http://www.integral-sk.com/wp-content/uploads/2013/01/abs4.jpg

SOLVED Question 3 The Double Integral Of Product Of Two Functions Is
https://cdn.numerade.com/ask_previews/1e6f325a-c71e-4b14-90b3-8107a399ee44_large.jpg
Let f and g be continuous increasing functions defined on 0 1 Prove that left int 0 1 f x dx right left int 0 1 g x dx right le int 0 1 f x g x dx This looks like A a Problem 2 i Starting from the inequality xy xp p yq q where x y p q 0 and 1 p 1 q 1 deduce Holder s integral inequality for continuous functions f t g t on a b b Z
In this note we establish new integral inequalities involving two functions and their derivatives The discrete analogues of the main results are also given Key words and phrases Integral The following inequality is a generalization of Minkowski s inequality C12 4 to double integrals In some sense it is also a theorem on the change of the order of iterated integrals but equality is

Finding The Product Of 2 Functions Algebra Study
https://study.com/cimages/videopreview/videopreview-full/sk55vytnok.jpg

Troubleshooting Evaluating A Trigonometric Integral Algebraically
https://mathvault.ca/wp-content/uploads/trig-integrals.jpg?x91276
integral of product of two functions inequality - In mathematical analysis H lder s inequality named after Otto H lder is a fundamental inequality between integrals and an indispensable tool for the study of L spaces The numbers p and q above are said to be H lder conjugates of each other The special case p q 2 gives a form of the Cauchy Schwarz inequality H lder s inequality holds even if fg 1 is infinite the right hand side also being infinite in that case Conversely if f is in L and g is in L th