if in the expansion of 2 x 1 4 x n Solution Verified by Toppr Given 2x 1 4x n General term of given expansion T r 1 n r 0nCr 2x n r 1 4x r When r 0 we get first term T 1 nC0 2x n T 1 nC0 2 nx When r 1 we get second term T 2 nC1 2x n 1 1 4x T 2 nC1 2 nx x 2x T 2 nC1 2 nx 3x As given Sum of coefficient of both term is 36 nC0 nC1 36 1 n 36
Free online series calculator allows you to find power series expansions of functions providing information you need to understand Taylor series Laurent series Puiseux series and more Mathematics Greatest Binomial Coefficients Question If in the expansion of 2x 1 4x T 3 T 2 7 and the sum of the coefficients of 2nd and 3rd term is 36 then the value of x is A 1 3 B 1 2 C 1 3 D 1 2 Solution Verified by Toppr Was this answer helpful 9 Similar Questions Q 1
if in the expansion of 2 x 1 4 x n
if in the expansion of 2 x 1 4 x n
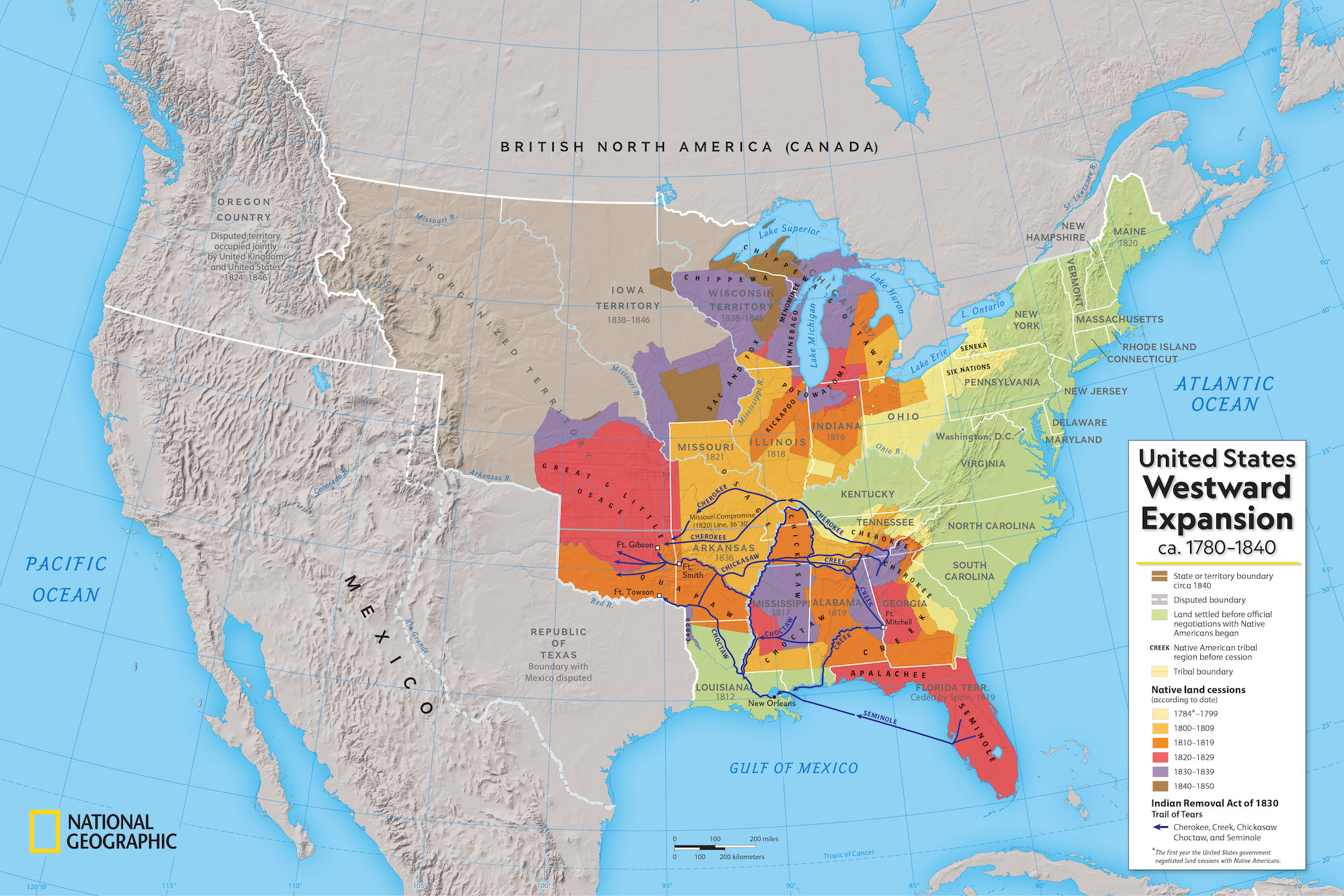
https://images.nationalgeographic.org/image/upload/v1638892465/EducationHub/photos/united-states-westward-expansion.jpg

Question Video Finding The Coefficient Of A Certain Term In A Binomial
https://media.nagwa.com/254128151254/en/thumbnail_l.jpeg

Binomial Expansion With 3 Terms YouTube
https://i.ytimg.com/vi/-hu_WT1CP9A/maxresdefault.jpg
CBSE Class 11 science Answered If in the expansion of 2 x 1 4 x n T3 T2 7 and the sum of the coefficients of 2nd and 3rd terms is 36 then the value of x is to calculate the answer The binomial theorem is also known as the binomial expansion which gives the formula for the expansion of the exponential power of a binomial expression Binomial expansion of x y n by using the binomial theorem is as follows x y n n C 0 x n y 0 n C 1 x n 1 y 1 n C 2 x n 2 y 2 n C n 1 x 1 y n 1 n C n x 0 y n
The coefficient of x 2 in the expansion of 1 x 5 n is 3 5 i Find the value of n ii With this value of n find the term independent of x in the expansion 1 x 5 n 2 3 x 2 So the answer is 3 3 3 3 2 x 3 x 2 3 x 3 we are replacing a by 3 and b by x in the expansion of a b 3 above Generally It is of course often impractical to write out Pascal s triangle every time when all that we need to know are the entries on the nth line Clearly the first number on the nth line is 1
More picture related to if in the expansion of 2 x 1 4 x n

If The First Three Terms In The Expansion Of 1 Ax n In Ascending
https://dwes9vv9u0550.cloudfront.net/images/4457894/6ff4e551-ed47-4988-ab1b-fffb8e70069b.jpg
EXPANSION JapaneseClass jp
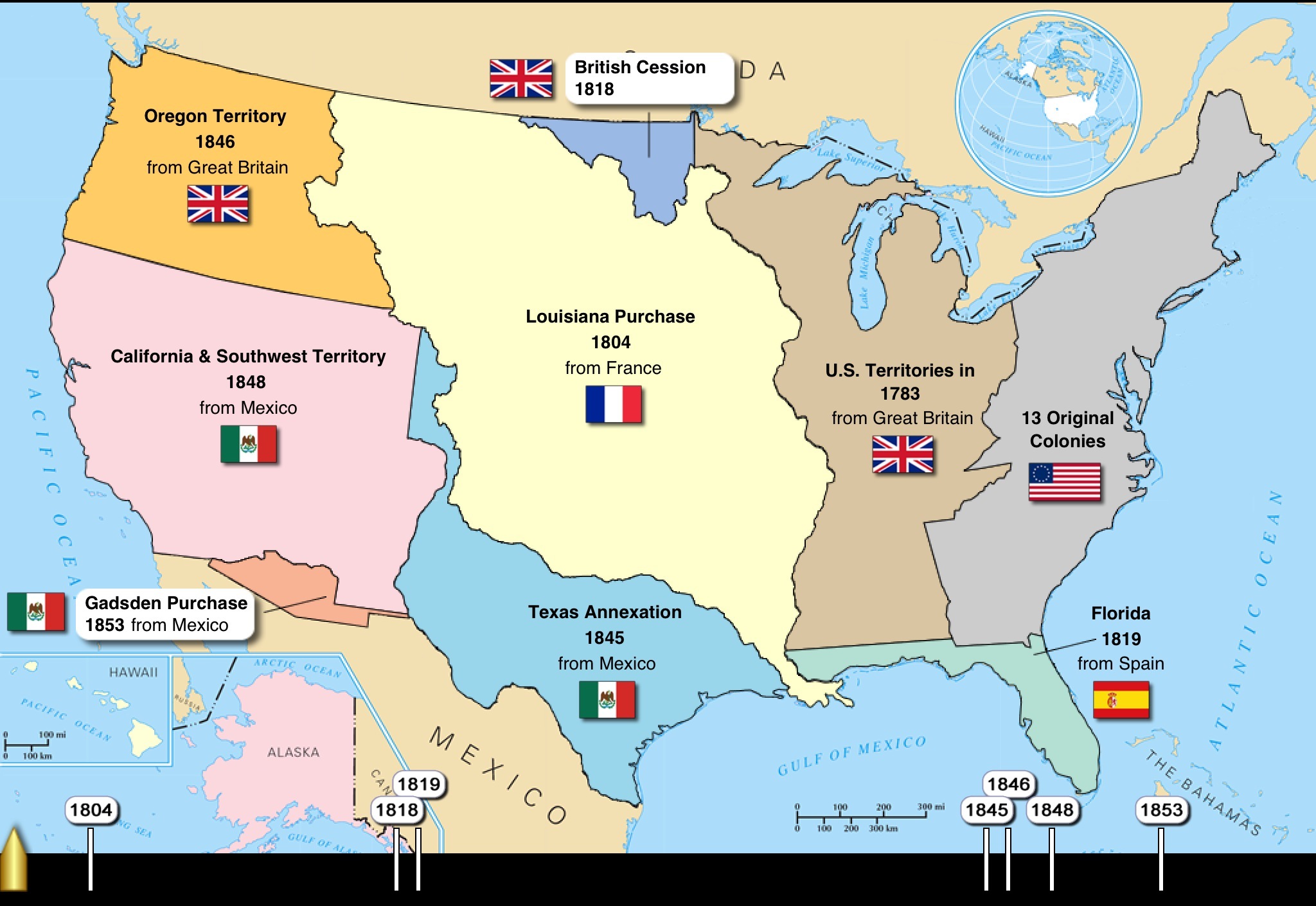
https://cdn.quotesgram.com/img/1/66/837362914-US_20Territorial_20Expansion_20Map.jpg

If The Coefficients Of X 7 X 8 In The Expansion Of 2 X 3 n Are
https://dwes9vv9u0550.cloudfront.net/images/9667380/f4c56489-b8a5-4f2e-a62f-b40042661637.jpg
The binomial theorem or binomial expansion is a result of expanding the powers of binomials or sums of two terms The coefficients of the terms in the expansion are the binomial coefficients binom n k kn Definition binomial A binomial is an algebraic expression containing 2 terms For example x y is a binomial We sometimes need to expand binomials as follows a b 0 1 a b 1 a b a b 2 a 2 2 ab b 2 a b 3 a 3 3 a 2b 3 ab 2 b 3 a b 4 a 4 4a 3b 6a 2b 2 4ab 3 b 4
This formula says x y n n C 0 0 x n y 0 n C 1 1 x n 1 y 1 n C 2 2 x n 2 y 2 n C 3 3 x n 3 y 3 n C n 1 n 1 x y n 1 n C n n x 0 y n Here we use nC k k formula to calculate the binomial coefficients which says n C k k n n k k Maths Question If the expansion of 1 x x2 n be a0 a1x a2x2 arxr a2nx2n show that a0 a3 a6 a1 a4 a7 a2 a5 a8 3n 1 Solution Verified by Toppr Given 1 x x2 n a0 a1x a2x2 a3x3 a4x4 1 Let 2 3 be the cube root unity then 1 2 0 and 3 1 4 5 2

Binomial Expansion Finding A Term Or Coefficient Tutorial 3
https://i.ytimg.com/vi/ktDy4ExrctQ/maxresdefault.jpg

Binomial Expansion Formula For 1 Plus X Whole Power N
https://www.onlinemath4all.com/images/x1plusxwholepowern.png.pagespeed.ic.axYIBwNCfa.png
if in the expansion of 2 x 1 4 x n - 3 Answers Sorted by 34 Well as I understand it we could write the binomial expansion as 1 x n k 0n n k 1n k x k 1 x n k 0 n n k 1 n k x k n 0 1n x 0 n 1 1n 1 x n 2 1n 2 x 2 n 3 1n 3 x 3 n 0 1 n x 0 n 1 1 n 1 x n 2 1 n 2 x 2 n 3 1 n 3 x 3