double angle identities trigonometry The double angle formulas are summarized as follows sin 2 2sin cos cos 2 cos2 sin2 1 2sin2 2cos2 1 tan 2 2tan 1 tan2 How to Given the tangent of an angle and the quadrant in which it is located use the double angle formulas to find the exact value
Exercise 7 3 1 Show cos 2 cos2 sin2 by using the sum of angles identity for cosine Answer For the cosine double angle identity there are three forms of the identity stated because the basic form cos 2 cos2 sin2 can be rewritten using the Pythagorean Identity Cos 2 cos2 sin2 2cos2 1 1 2sin2 The double angle identity for the sine function uses what is known as the cofunction identity Remember that in a right triangle the sine of one angle is the same as the cosine of its complement which is
double angle identities trigonometry
double angle identities trigonometry
http://4.bp.blogspot.com/-ZX1JxQfXTkE/T6132Y3eWnI/AAAAAAAAHDE/Lz4trGiGAlM/s1600/Slide3.JPG
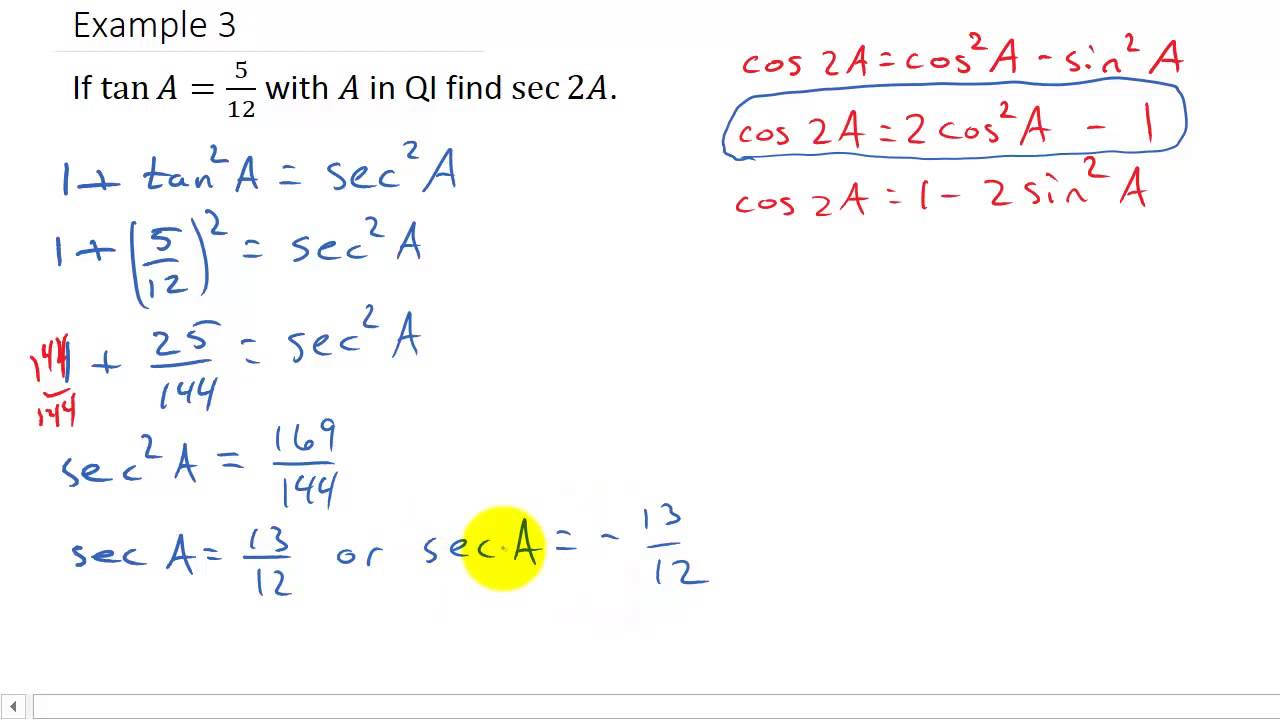
Trigonometry Double Angle Identities Example 3 YouTube
https://i.ytimg.com/vi/YlVw-XVMP5I/maxresdefault.jpg
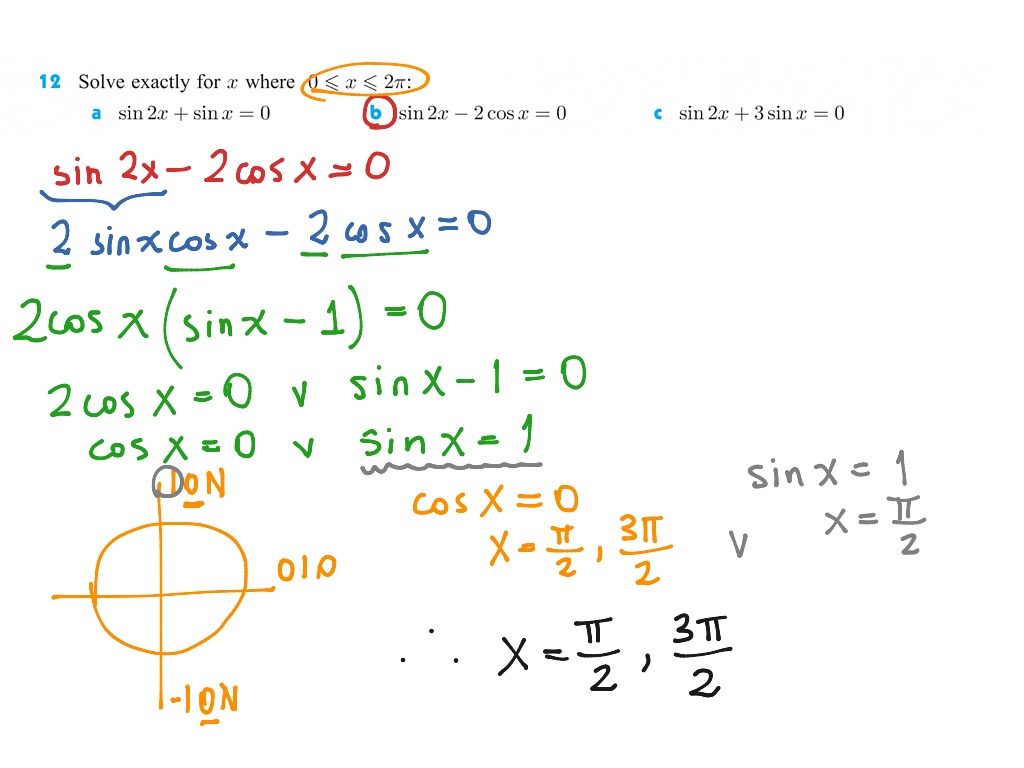
Double Angle Identities Ex 9 Math Trigonometry Double Angle
https://showme1-9071.kxcdn.com/2020/04/20/07/5CdRoSO_ShowMe_last_thumb.jpg
Double Angle Formulas begin align sin 2x 2 sin x cos x cos 2x cos 2 x sin 2 x 2 cos 2 x 1 1 2 sin 2 x tan 2x frac 2 tan x 1 tan 2 x end align From these formulas we also have the following identities Trigonometry Trigonometric Identities Formulas expressing trigonometric functions of an angle 2x in terms of functions of an angle x sin 2x 2sinxcosx 1 cos 2x cos 2x sin 2x 2 2cos 2x 1 3 1 2sin 2x 4 tan 2x 2tanx 1 tan 2x
This trigonometry video tutorial provides a basic introduction to the double angle identities of sine cosine and tangent It explains how to derive the double angle formulas from the The trigonometric double angle formulas give a relationship between the basic trigonometric functions applied to twice an angle in terms of trigonometric functions of the angle itself From these formulas we also have the following identities
More picture related to double angle identities trigonometry
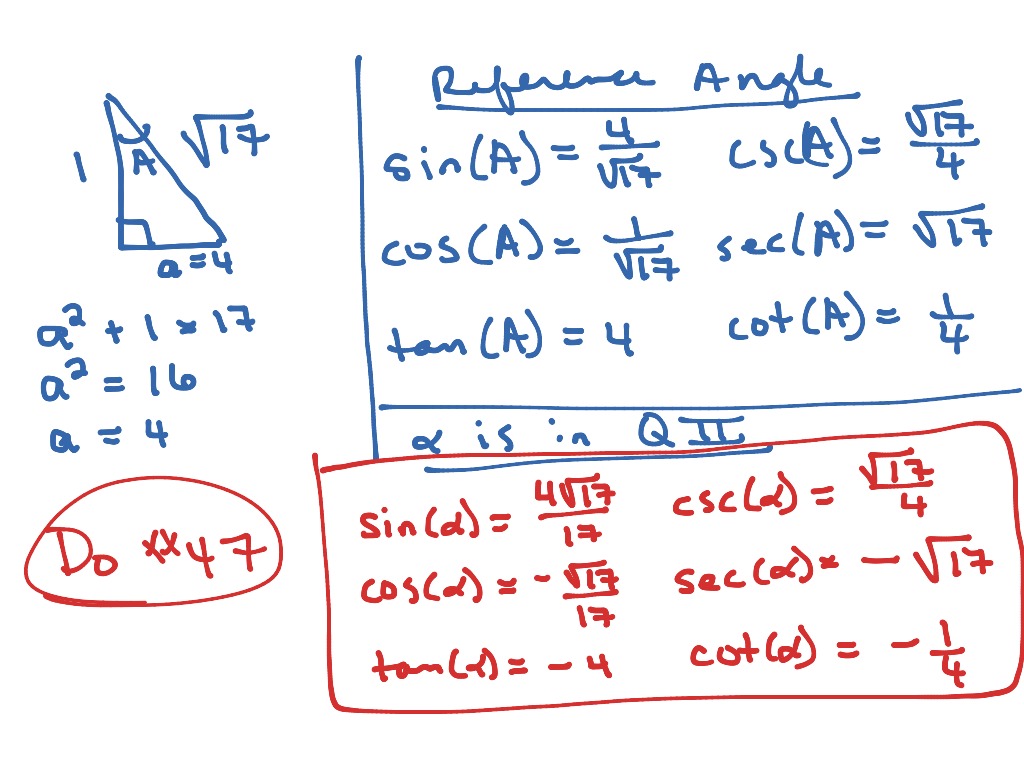
Section 6 4 Double Angle Identities 31 Min Math Trigonometry
https://showme0-9071.kxcdn.com/files/88218/pictures/thumbs/1411892/last_thumb1393146675.jpg

Inverse Trig Functions With Double Angle Formulas And Half Angle
https://i.ytimg.com/vi/waDbbpib9cM/maxresdefault.jpg

Double Angle Identities Trigonometry School Yourself
https://d1hepzhkxkhmpd.cloudfront.net/sy/2016-07-31/trigonometry/trig_identities_double/module_screenshot_big.jpg
The double angle formulas are summarized as follows sin 2 2 sin cos cos 2 cos2 sin2 1 2 sin2 2cos2 1 tan 2 2 tan 1 tan2 Page ID Simplifying trigonometric functions with twice a given angle These identities are significantly more involved and less intuitive than previous identities By practicing and working with these advanced identities your toolbox and fluency substituting and proving on your own will increase
The cosine double angle formula tells us that cos 2 is always equal to cos sin For example cos 60 is equal to cos 30 sin 30 We can use this identity to rewrite expressions or solve problems See some examples in this video Created by Sal Khan Double angle identities are trigonometric identities used to rewrite trigonometric functions such as sine cosine and tangent that have a double angle such as 2 These identities are derived using the angle sum identities We have a total of three double angle identities one for cosine one for sine and one for tangent

Trigonometry Finding Exact Value Using Double Angle Identities YouTube
https://i.ytimg.com/vi/i3B8WW8tMaw/maxresdefault.jpg

Trigonometry Double Angle Identities Expii
https://d20khd7ddkh5ls.cloudfront.net/img_0279_2.jpg
double angle identities trigonometry - This trigonometry video tutorial provides a basic introduction to the double angle identities of sine cosine and tangent It explains how to derive the double angle formulas from the