a 0 1 2 3 4 5 then the number of subsets of a is A has total 32 subsets but one of them is A itself Proper subsets of A are 32 1 31
Given a set 1 2 n 1 2 n how would one go about finding the number of subsets that contain the number 2 for example If the number of 5 elements subsets of the set A a1 a2 a20 of 20 distinct elements is k times the number of 5 elements subsets containing a4 then k is View Solution Q 4
a 0 1 2 3 4 5 then the number of subsets of a is
a 0 1 2 3 4 5 then the number of subsets of a is
https://www.coursehero.com/qa/attachment/13681651/

Using A Venn Diagram Find The Number Of A Subset Of A Given Set And
https://s3mn.mnimgs.com/img/shared/content_ck_images/ck_617a3dcde9855.jpg

How To Count Subsets Mathematics Of Machine Learning
https://assets.tivadardanka.com/2022_03_number_of_subsets_featured_d366fbdea9.png
Solution Verified by Toppr A 1 2 3 4 Number of elements in A n 3 Total number of subsets of A 2n 23 8 Except for set A and null set all other sets are proper sets Addition principle Number of ways of choosing n objects or m objects is done in n m ways Calculation Number of elements in the given set is n A 10 Number of subset containing two elements is same as choosing two elements out of the given 10 elements It is given by 10 C 2 45
The number of proper subsets of any set is given by the formula 2 n 1 where n is the number of elements in the set A proper subset of a set A is a subset of A that is not equal to A In other words if B is a proper subset of A then all elements of B are in A but A contains at least one element that is not in B The number of proper subsets is 2n 1 where n is the count of distinct numbers in a set
More picture related to a 0 1 2 3 4 5 then the number of subsets of a is

Solved Calculate The Number Of Subsets And The Number Of Chegg
https://media.cheggcdn.com/study/0ba/0ba83334-65fd-4556-a3c2-f6d33f0ce8ed/image.png
Solved List All Subsets Or Determine The Number Of Subsets As
https://www.coursehero.com/qa/attachment/12697032/
If Number Of Subsets Of Set A Is 3968 More Than The Number Of Subset Of
https://search-static.byjusweb.com/question-images/aakash_pdf/99996928624-0-0
Solution Verified by Toppr Set A has 3 elements as 3 4 are combined together as one element Number of non empty proper subsets of a set with n elements is 2n 2 So Number of non empty proper subsets of a set with 3 elements is 23 2 8 2 6 Was this answer helpful 4 Similar Questions Q 1 Solution Total number of subsets of A is 2n A 26 64 Number of subsets of A which contain at least two elements is 64 6C 0 6C 1 64 1 6 57 KCET 2020 If A 1 2 3 4 5 6 then the number of subsets of A which contain at least two elements is A 64 B 63 C 57 D 58 Check Answer and So
For example for a set A 1 2 the subsets will be 1 2 1 2 If a set includes n elements the number of subsets for that set is 2 and the correct subset is 2 1 Using the same formula the number of subsets of set A will be 16 and the elements of the same are listed above Correct Answer Option 3 15 Concept If A is a non empty set such that n A m then number of proper subsets of A is given by 2 m 1 Calculation Given A 1 2 3 4
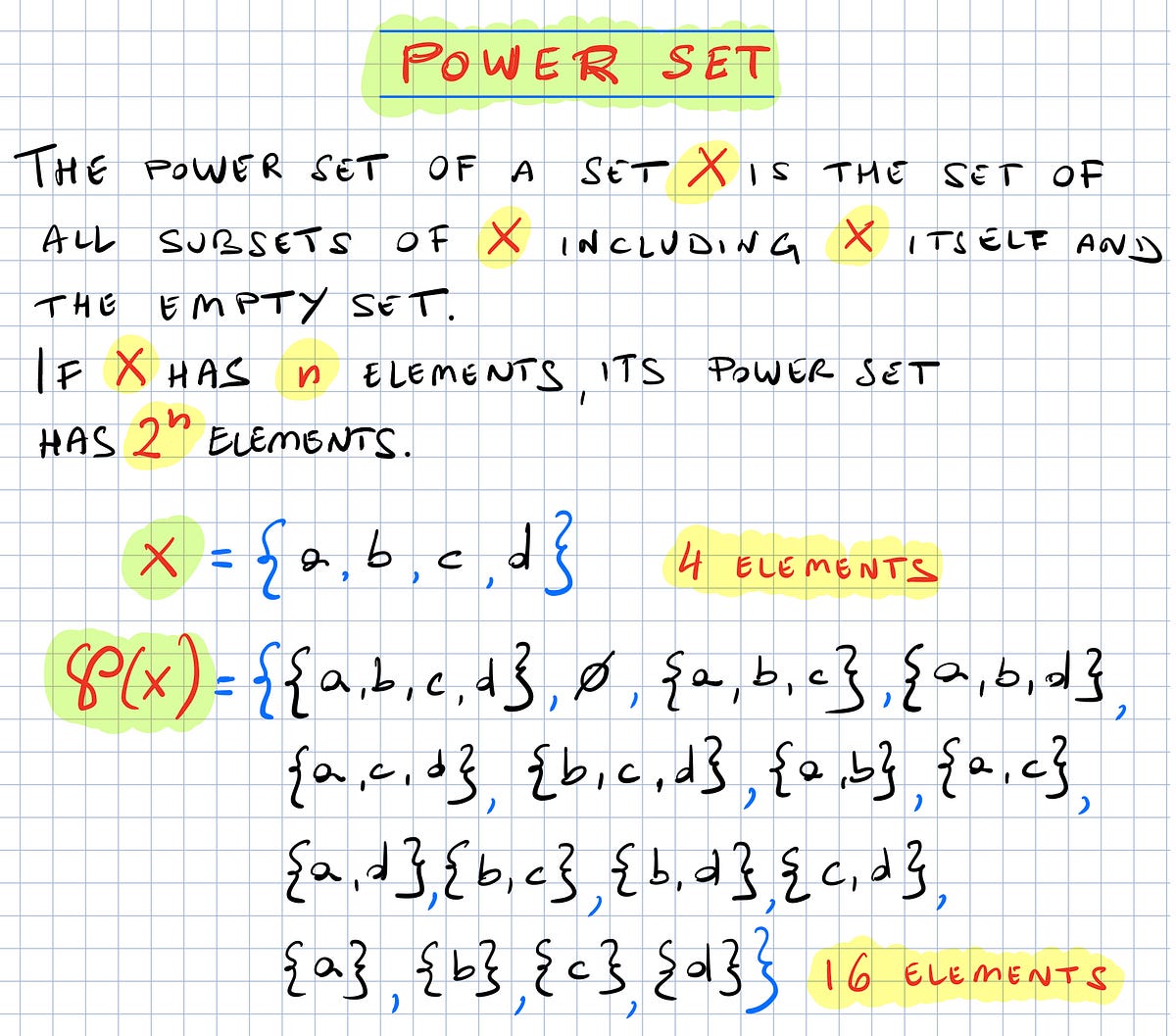
Power Set The Set Of All Subsets Of A Given Set By Michele Diodati
https://miro.medium.com/v2/resize:fit:1200/1*J7-VQ7WYiAC3mUBiPvwqYQ.jpeg

Two Finite Sets Have M And N Elements The Total Number Of Subsets Of
https://hi-static.z-dn.net/files/d25/0af25a15f80e75ee2091da7de8c846c3.jpg
a 0 1 2 3 4 5 then the number of subsets of a is - The number of proper subsets of any set is given by the formula 2 n 1 where n is the number of elements in the set