5 examples of rational algebraic expressions 1 Addition and Subtraction of Rational Expressions a Addition and Subtraction of Rational Expressions With the Same Denominator b Addition and Subtraction of Rational Expressions With Different Denominators 2 Multiplication of Rational Expressions Using Cancellation Method in Multiplying Rational Expressions 3
We now need to look at rational expressions A rational expression is nothing more than a fraction in which the numerator and or the denominator are polynomials Here are some examples of rational expressions frac 6 x 1 hspace 0 25in frac z 2 1 z 2 5 hspace 0 25in frac m 4 This topic covers Simplifying rational expressions Multiplying dividing adding subtracting rational expressions Rational equations Graphing rational functions including horizontal vertical asymptotes Modeling with rational functions Rational inequalities Partial fraction expansion
5 examples of rational algebraic expressions
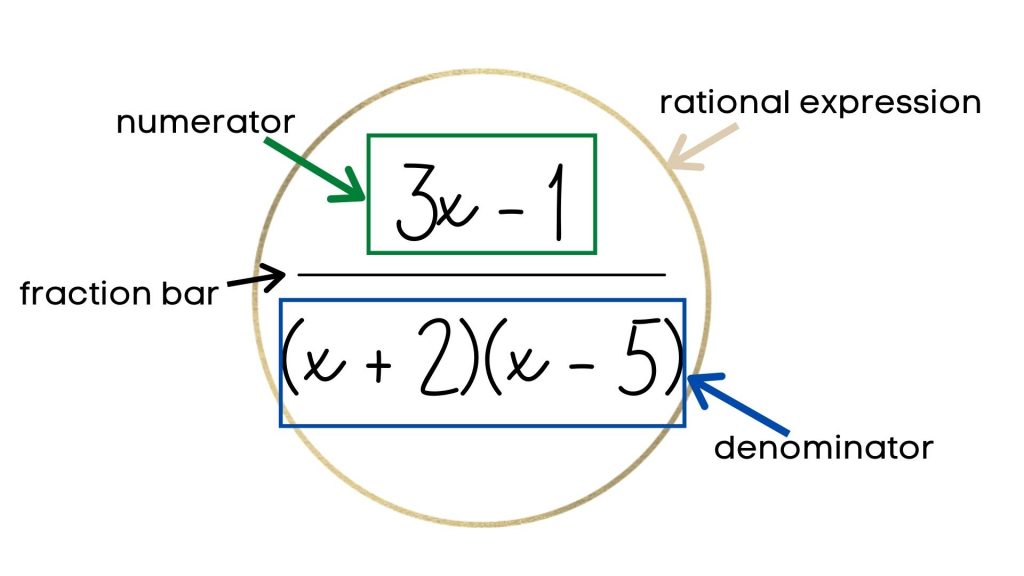
5 examples of rational algebraic expressions
https://intomath.org/wp-content/uploads/2021/02/rational-expression-1-1024x576.jpg

Solving Word Problem Involving Rational Algebraic Expression YouTube
https://i.ytimg.com/vi/151EYpDvcrA/maxresdefault.jpg
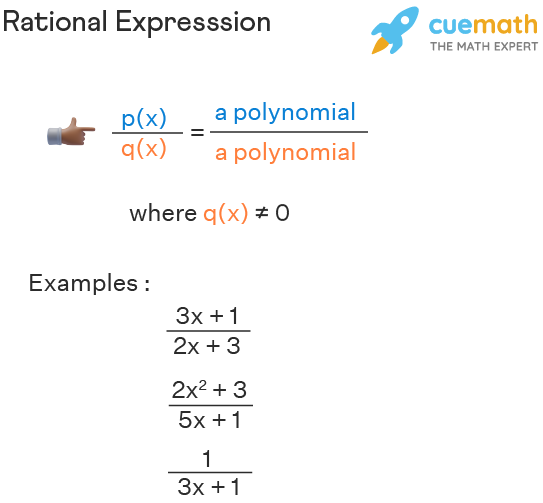
Rational Expression Simplification Add Multiply Share 2022
https://d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/rational-expression-1655444183.png
Here is a set of practice problems to accompany the Rational Expressions section of the Preliminaries chapter of the notes for Paul Dawkins Algebra course at Lamar University The quotient of two polynomials is a rational expression The denominator of a rational expression can never have a zero value The following are examples of rational expressions The last example 6 x 5 could be expressed as Therefore it satisfies the definition of a rational expression
These are examples of rational expressions 1 x x 5 x 2 4 x 4 x x 1 2 x 3 x 6 Notice that the numerator can be a constant and that the polynomials can be of varying degrees and in multiple forms Rational expressions and undefined values Consider the rational expression 2 x 3 x 2 A rational expression is an algebraic expression that can be written as the quotient of two polynomials Examples 1 4 are rational expressions Example PageIndex 1
More picture related to 5 examples of rational algebraic expressions
Simplifying Rational Algebraic Expressions Exercises YouTube
https://i.ytimg.com/vi/c8Se_HdiquE/maxresdefault.jpg

Simplify The Rational Expression 5 YouTube
https://i.ytimg.com/vi/YXA617JzjVs/maxresdefault.jpg

ILLUSTRATING RATIONAL ALGEBRAIC EXPRESSIONS GRADE 8 MATHEMATICS Q1
https://i.ytimg.com/vi/qQCbPRBOnIc/maxresdefault.jpg
Quiz Unit test About this unit Rational expressions bring together what we know from fractions with our polynomial skills They arise in a variety of contexts involving rates particularly when there are unknowns or changing values in the denominator Simplifying Rational Expressions The quotient of two polynomial expressions is called a rational expression We can apply the properties of fractions to rational expressions such as simplifying the expressions by canceling common factors from the numerator and the denominator To do this we first need to factor both the numerator and
Similarly we define a rational expression or algebraic fraction frac P Q as the quotient of two polynomials P and Q where Q 0 Some examples of rational expressions follow The example frac x 3 x 5 consists of linear expressions in both the numerator and denominator Here are some examples of rational expressions x 1 x 2 5 x 3 3x 2 5 4x 2 etc Note that if one of the numerator and denominator is NOT a polynomial then the fraction is NOT called as a rational expression Restrictions of Rational Expression Dividing a number by 0 is not possible
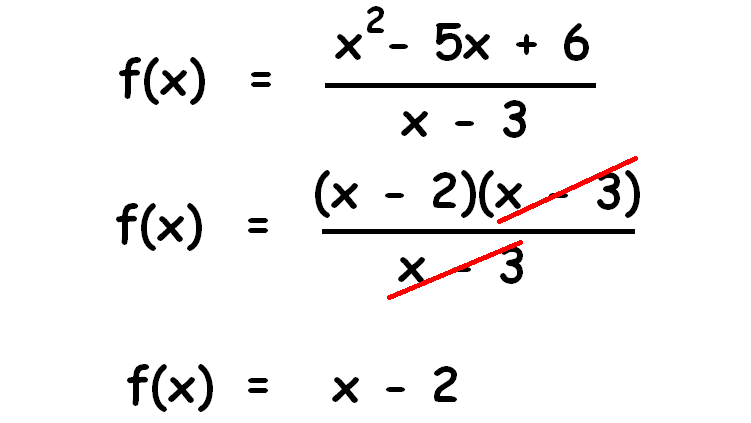
Generating Equivalent Algebraic Expressions
https://www.onlinemath4all.com/images/simplifyingrationalfunctions1.png
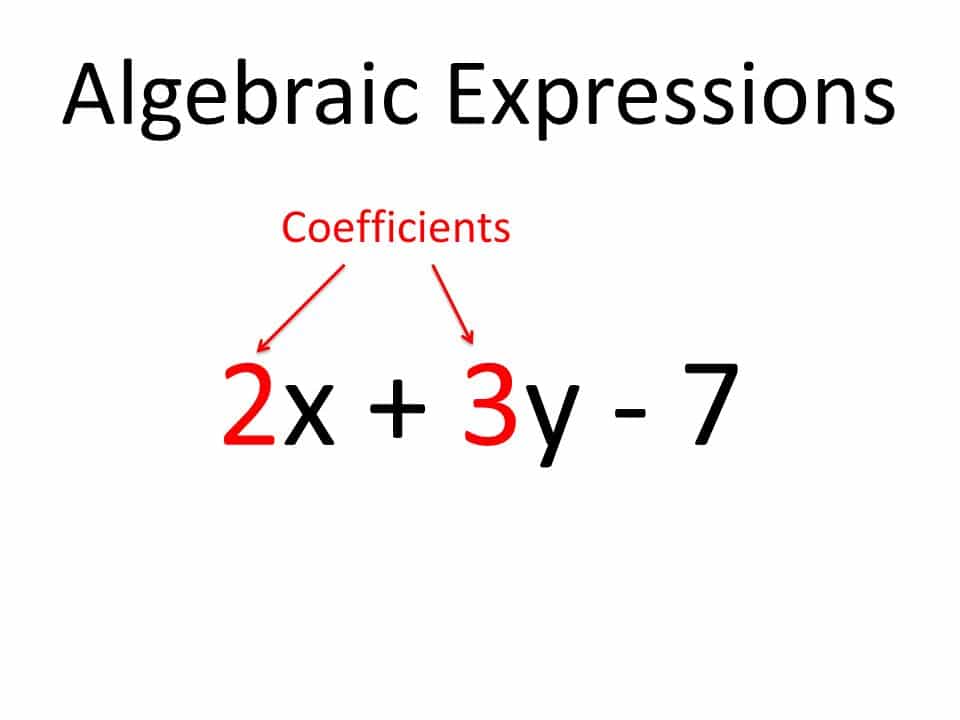
Algebraic Expression Examples Expressions Education Is Around
https://educationisaround.com/wp-content/uploads/2020/12/images-6.jpg
5 examples of rational algebraic expressions - Problem 1 Add The numerator should be expanded and simplified The denominator should be either expanded or factored 5 x x 3 4 x 2 Check Least common denominators Numerical fractions Sometimes the denominators of two fractions are different but have some shared factors For example consider 3 4 1 6