1 1 2 1 2 3 series formula The series sum limits k 1 n k a 1 a 2 a 3 a cdots n a gives the sum of the a text th powers of the first n positive numbers where a and n are positive integers Each of these series can be calculated through a closed form formula
f n 1 1 1 2 1 3 1 n is using Harmonics Numbers f n H n the n th harmonic number A x This result holds if f x has continuous derivatives of order n at last If lim R 0 the infinite series obtained is called n n Taylor series for f x about x a If a 0 the series is often called a Maclaurin series
1 1 2 1 2 3 series formula

1 1 2 1 2 3 series formula
https://1.bp.blogspot.com/-vjNTKshgPec/WDuId6M7DXI/AAAAAAAAFB0/mj-BUNqAw9MfOdI6dtsBfYrdT99mmZJxQCPcB/s1600/NR%2BWDC%2B2016%2BAbuDGP%2B2.jpg

1 2 3
https://peda.net/orivesi/oriveden-kielipolku/veeja/ejave3/numerot2/1-2-3:file/photo/061f944575d2da88c7f003f96a812ce1ee8e037d/1-2-3.jpg

Taylor Series Formula
https://sigmatricks.com/wp-content/uploads/2021/02/Taylor-Series.jpg
Your sum is equal to n n 1 2 n 1 12 n n 1 4 This is obtained by writing it as a sum and using the fact that the sum of the first n consecutive squares is n n 1 2n 1 6 and the sum of the first n positive ints is n n 1 2 1 if you can find a nicer form of the formula Learn the general form of the arithmetic series formula and the difference between an arithmetic sequence and an arithmetic series Discover the partial sum notation and how to use it to calculate the sum of n terms
Find the Sum of the Infinite Geometric Series 16 4 1 1 4 16 4 1 1 4 Free sum of series calculator step by step solutions to help find the sum of series and infinite series In example to get formula for 1 2 2 2 3 2 n 2 they express f n as f n an 3 bn 2 cn d also known that f 0 0 f 1 1 f 2 5 and f 3 14 Then this values are inserted into fu
More picture related to 1 1 2 1 2 3 series formula

1 2 3
https://media.lekia.se/lekia/images/image-1-2-3-2021-03-09-145554641/555/555/2/1-2-3.jpg
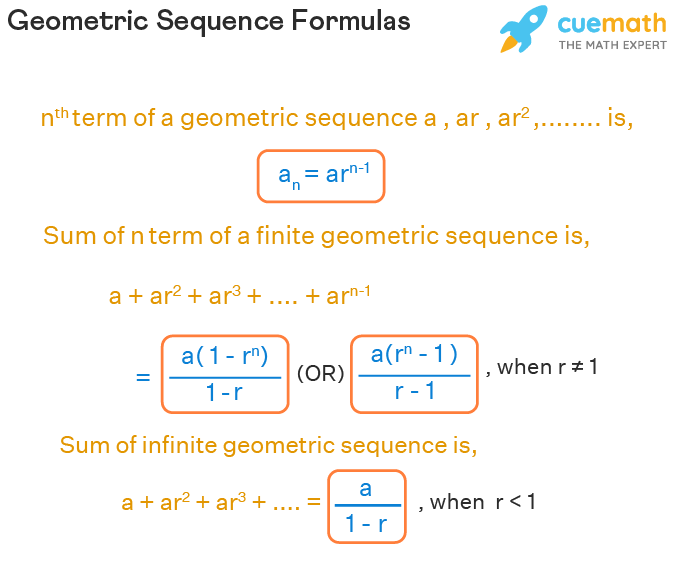
Geometric Sequence Formulas What Is Geometric Sequence Formula
https://d138zd1ktt9iqe.cloudfront.net/media/seo_landing_files/geometric-sequence-formulas-1650451104.png
1 1 2 BSPT TEMP PRES SAFETY VALVE 2BAR Pressure Relief Valve
https://cdn.pimber.ly/public/asset/raw/5ee8a13150af727432f87049/4d07eba4/609ce9bbc9a2c2001862456c/34836671/IFT-M316-20610W-large1425.png?w=3840&q=100
1 1 2 1 3 1 n n 1 1 1 2 1 3 1 n n 1 where is Euler s constant and is the digamma function Of course one reason for creating the digamma function is to make formulae like this true Case 1 Sum of n Natural Numbers 1 2 3 4 n This arithmetic series represents the sum of n natural numbers Let us try to calculate the sum of this arithmetic series The difference between the sum of n natural numbers and sum of n 1 natural numbers is n i e S n S n 1 n
A partial sum of an infinite series is a finite sum of the form k n 1an a1 a2 a3 ak To see how we use partial sums to evaluate infinite series consider the following example Suppose oil is seeping into a lake N 1 1 2 1 4 1 8 1 2 log2 n The 2nd factor is called a partial sum of the geometric series which converges meaning that as we approach the infinity it will approach a constant Therefore it is 1 when you multiply this by n you get n

PDF Set Series Combinations Additions Etc
https://i1.rgstatic.net/publication/368191114_Set_Series_Combinations_Additions_Etc/links/63dce85962d2a24f92f4ec43/largepreview.png

Proram Induction Kit Bmw 1 2 3 Series N20 Engines Rennsport
http://rennsportperformance.co.uk/cdn/shop/products/54f11e67-47d1-4d01-8ee3-b204edc5125a__76543.webp?v=1681424478
1 1 2 1 2 3 series formula - Sum of the series 1 1 2 2 3 3 n n using recursion in C In this problem we are given a number n which defines the nth terms of the series 1 1 2 2 3 3 n n Our task is to create a program that will find the sum of the series